Find the Line Equation Through Points (5,0) and (1/2,4.5): Coordinate Method

Find the equation of the line passing through the two points (5,0),(12,412) (5,0),(\frac{1}{2},4\frac{1}{2})

❤️ Continue Your Math Journey!

We have hundreds of course questions with personalized recommendations + Account 100% premium

Step-by-step video solution

Watch the teacher solve the problem with clear explanations
00:08 Let's find the algebraic representation of the function, shall we?
00:12 First, we're going to use a formula to determine the slope using two points. Ready?
00:18 At each point, remember, the left number is the X-axis and the right is the Y-axis. Easy, right?
00:26 Now, let's substitute these points into the formula with the data given, and find the slope. Let's do it!
00:42 Great! This number is the line's slope.
00:49 Next, we're going to use the line equation.
00:53 Substitute the point in the equation, following the data provided. You've got this!
00:59 Now, substitute the slope and solve for the intersection point, which we'll call 'B'. Keep going!
01:06 Let's isolate the intersection point, 'B'. Almost there!
01:12 And here is the intersection point with the Y-axis. Awesome job!
01:17 Now, plug this intersection point and the slope back into the line equation. You're doing amazing!
01:32 Let's tidy up the equation. Final steps!
01:39 And there you have it! That's the solution to our question. Well done!

Step-by-step written solution

Follow each step carefully to understand the complete solution
1

Understand the problem

Find the equation of the line passing through the two points (5,0),(12,412) (5,0),(\frac{1}{2},4\frac{1}{2})

2

Step-by-step solution

First, we will use the formula to find the slope of the straight line:

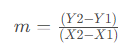
We replace the data and solve:

(0−4.5)(5−0.5)=−4.54.5=−1 \frac{(0-4.5)}{(5-0.5)}=\frac{-4.5}{4.5}=-1

Now, we know that the slope is −1 -1

 

We replace one of the points in the formula of the line equation:

y=mx+b y=mx+b

(5,0) (5,0)

0=−1×5+b 0=-1\times5+b

 0=−5+b 0=-5+b

b=5 b=5

Now we have the data to complete the equation:

y=−1×x+5 y=-1\times x+5

y=−x+5 y=-x+5

3

Final Answer

y+x=5 y+x=5

Practice Quiz

Test your knowledge with interactive questions

Look at the function shown in the figure.

When is the function positive?

xy-4-7

🌟 Unlock Your Math Potential

Get unlimited access to all 18 Linear Functions questions, detailed video solutions, and personalized progress tracking.

📹

Unlimited Video Solutions

Step-by-step explanations for every problem

📊

Progress Analytics

Track your mastery across all topics

🚫

Ad-Free Learning

Focus on math without distractions

No credit card required • Cancel anytime

More Questions

Click on any question to see the complete solution with step-by-step explanations

Equation of a Straight Line

Find the Line Equation Through Points (1/3,1) and (-1/3,2): Coordinate Geometry
Click to solve
Find the Line Equation Through Points (12,40) and (2,10): Step-by-Step
Click to solve