What data must be added so that the triangles are congruent? 65 65 65 5 5 5 8 8 8 5 5 5 8 8 8 A A A B B B C C C D D D E E E F F F

It is not possible to add data for the triangles to be congruent.

What data must be added so that the triangles are congruent? 41 41 41 39 39 39 7 7 7 9 9 9 9 9 9 7 7 7 A A A B B B C C C D D D E E E F F F

Data cannot be added for the triangles to be congruent.

SSA Congruence Rule Practice Problems & Solutions

Understanding Side, Side, Angle Congruence Rule

Complete explanation with examples

Fourth Congruence Theorem: Side-Side-Angle

Congruence in geometry refers to two figures that have the exact same shape and size, meaning they can perfectly overlap when placed on top of one another.

There are 4 criteria to determine that two triangles are congruent. In this article, we will learn to use the fourth criterion of congruence:

Fourth Congruence Theorem: Side-Side-Angle

In summary: SSA

It means that:
if two triangles have two pairs of equal sides and the angle opposite the larger of these two pairs is also equal, then the triangles are congruent.

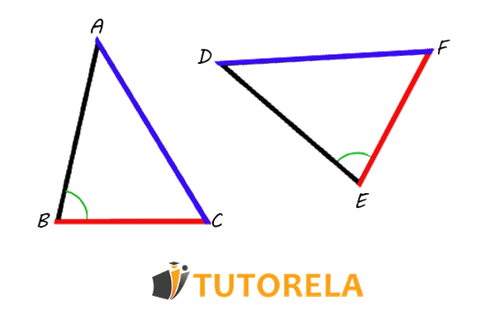
Recognizing the SSA Pattern:

In this criterion, you have two sides of a triangle and an angle that is not between them. However, unlike other congruence criteria, SSA can be ambiguous. Depending on the angle’s size and the relationship between the sides, multiple triangle configurations can arise.

The Ambiguity of SSA:

A key thing to remember is that the SSA criterion does not always lead to a unique triangle. When the angle is acute, two different triangles may satisfy the given side and angle conditions. This is referred to as the "ambiguous case" in trigonometry. It occurs because depending on the relative length of the sides, there may be two possible solutions, one solution, or no solution.

Flipped and Rotated Triangles:

Like with other triangle congruence criteria, flipping or rotating the triangle will not change its congruence. So, when matching triangles, always ensure that you are comparing corresponding sides and angles, even if the triangles are oriented differently.

Detailed explanation

Practice Side, Side, Angle Congruence Rule

Test your knowledge with 5 quizzes

Look at the triangles in the diagram.

Which of the statements is true?

Examples with solutions for Side, Side, Angle Congruence Rule

Step-by-step solutions included

Exercise #1

Look at the triangles in the diagram.

Determine which of the statements is correct.

Step-by-Step Solution

Let's consider that:

AC=EF=4

DF=AB=5

Since 5 is greater than 4 and the angle equal to 34 is opposite the larger side in both triangles, the angle ACB must be equal to the angle DEF

Therefore, the triangles are congruent according to the SAS theorem, as a result of this all angles and sides are congruent, and all answers are correct.

Answer:

All of the above.

Exercise #2

Look at the triangles in the diagram.

Which of the following statements is true?

Step-by-Step Solution

According to the existing data:

$EF=BA=10$ (Side)

$ED=AC=13$ (Side)

The angles equal to 53 degrees are both opposite the greater side (which is equal to 13) in both triangles.

(Angle)

Since the sides and angles are equal among congruent triangles, it can be determined that angle DEF is equal to angle BAC

Answer:

Angles BAC is equal to angle DEF.

Exercise #3

Look at the triangles in the diagram.

Which of the following statements is true?

Step-by-Step Solution

This question actually has two steps:

In the first step, you must define if the triangles are congruent or not,

and then identify the correct answer among the options.

Let's look at the triangles: we have two equal sides and one angle,

But this is not a common angle, therefore, it cannot be proven according to the S.A.S theorem

Remember the fourth congruence theorem - S.A.A
If the two triangles are equal to each other in terms of the lengths of the two sides and the angle opposite to the side that is the largest, then the triangles are congruent.

But the angle we have is not opposite to the larger side, but to the smaller side,

Therefore, it is not possible to prove that the triangles are congruent and no theorem can be established.

Answer:

It is not possible to calculate.

Exercise #4

Which of the triangles are congruent?

Step-by-Step Solution

Let's observe the angle in each of the triangles and note that each time it is opposite to the length of a different side.

Therefore, none of the triangles are congruent since it is impossible to know from the data.

Answer:

It is not possible to know based on the data.

Exercise #5

What data must be added so that the triangles are congruent?

Step-by-Step Solution

Let's consider that:

DF = AC = 8

DE = AB = 5

8 is greater than 5, therefore the angle DEF is opposite the larger side and is equal to 65 degrees.

That is, the figure we are missing is the angle of the second triangle.

We will examine which angle is opposite the large side AC.

ABC is the angle opposite the larger side AC so it must be equal to 65 degrees.

Answer:

Angle ABC equals 65.

Frequently Asked Questions

Everything you need to know about Side, Side, Angle Congruence Rule

What is the SSA congruence rule for triangles?

The SSA (Side-Side-Angle) congruence rule states that if two triangles have two pairs of equal sides and the angle opposite the larger of these two sides is also equal, then the triangles are congruent. This is also known as the fourth congruence theorem.

When does SSA not prove triangle congruence?

SSA does not prove congruence when the given angle is opposite the shorter side, or when the angle is acute and creates an ambiguous case. In the ambiguous case, two different triangles can satisfy the same SSA conditions, making congruence impossible to determine.

How do you determine which side is longer in SSA problems?

You can determine the longer side by: 1) Direct measurement if numerical values are given, 2) Using the fact that sides opposite larger angles are longer, 3) Recognizing that the side opposite a 90° or obtuse angle is always the longest side in a triangle.

What is the ambiguous case in SSA triangle congruence?

The ambiguous case occurs when you have two sides and an acute angle opposite the shorter side. In this situation, two different triangles may satisfy the given conditions, or sometimes no triangle exists at all, making it impossible to prove congruence using SSA.

Can SSA be used if the angle is between the two given sides?

No, if the angle is between the two given sides, you should use SAS (Side-Angle-Side) congruence instead. SSA specifically requires that the angle be opposite one of the given sides, and it must be opposite the longer of the two sides.

How do you write a formal proof using SSA congruence?

To write an SSA proof: 1) State the two pairs of equal sides, 2) Identify which side is longer in each triangle, 3) Show that the angles opposite the longer sides are equal, 4) Conclude that the triangles are congruent by SSA, 5) List all corresponding equal parts.

What are the requirements for SSA triangle congruence?

SSA requires three conditions: 1) Two sides of one triangle equal two sides of another triangle, 2) An angle in one triangle equals an angle in the other triangle, 3) The equal angles must be opposite the longer sides in both triangles.

Why is SSA congruence less reliable than other congruence theorems?

SSA is less reliable because it can create ambiguous situations where multiple triangles satisfy the same conditions. Unlike SAS, ASA, or SSS which always produce unique triangles, SSA requires the additional condition that the angle be opposite the larger side to ensure congruence.

Practice by Question Type

Choose your preferred learning style and practice format for fraction problems

Ascertaining whether or not the triangles are congruent Identifying and defining elements