Division in a given ratio - Examples, Exercises and Solutions

Question Types:
Ratio: Impact of a radius change on the area of a circleRatio: Using additional geometric shapesRatio: Calculate the ratio between radii of two circles

What is division in a given ratio?

Division in a given ratio means splitting a total quantity into parts that maintain a specific proportional relationship, based on the ratio provided.
In a division according to a given ratio, we will have a defined quantity that we must divide according to said ratio. The process ensures that the ratio between the parts stays consistent, regardless of the total amount being divided. This concept is frequently used in various scenarios, such as dividing an inheritance, sharing resources, or solving problems in geometry.

Let's use an Example:

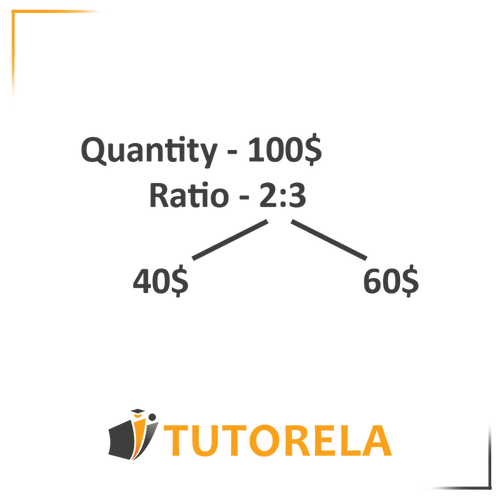
We want to divide 100100 Dollars in a 2:32:3 ratio.
So, the quantity is 100100 , and the ratio provided is 2:32:3 .

In order to do so, let's follow there simple steps:

  1. Add the parts of the ratio. In our case: 2+3=52 + 3 = 5 .
    Now we know that we need to divide the quantity to 55 .
  2. Divide the total amount by 55 . In our case: 100:5=20100:5=20
    So we get 2020 Dollars per part.
  3. Multiply each of the ratio side by the part.
    So: 20⋅2=4020\cdot2=40 , 3⋅20=603\cdot20=60 .

And so the 100100 Dollars is divided into 4040 Dollars and 6060 Dollars , maintaining the 2:32:3 ratio.

Detailed explanation

Practice Division in a given ratio

Question 1

There are two circles.

One circle has a radius of 4 cm, while the other circle has a radius of 10 cm.

How many times greater is the area of the second circle than the area of the first circle?

Question 2

Given the rectangle ABCD

AB=X the ratio between AB and BC is equal to\( \sqrt{\frac{x}{2}} \)

We mark the length of the diagonal \( A \) with \( m \)

Check the correct argument:

XXXmmmAAABBBCCCDDD

Question 3

Given the rectangle ABCD

AB=X

The ratio between AB and BC is \( \sqrt{\frac{x}{2}} \)

We mark the length of the diagonal A the rectangle in m

Check the correct argument:

XXXmmmAAABBBCCCDDD

Question 4

There are 18 balls in a box, \( \frac{2}{3} \) of which are white.

How many white balls are there in the box?

Question 5

In a box there are 28 balls, \( \frac{1}{4} \) of which are orange.

How many orange balls are there in the box?

Examples with solutions for Division in a given ratio

Exercise #1

There are two circles.

One circle has a radius of 4 cm, while the other circle has a radius of 10 cm.

How many times greater is the area of the second circle than the area of the first circle?

Video Solution

Step-by-Step Solution

The area of a circle is calculated using the following formula:

where r represents the radius.

Using the formula, we calculate the areas of the circles:

Circle 1:

π*4² =

π16

Circle 2:

π*10² =

π100

To calculate how much larger one circle is than the other (in other words - what is the ratio between them)

All we need to do is divide one area by the other.

100/16 =

6.25

Therefore the answer is 6 and a quarter!

Answer

614 6\frac{1}{4}

Exercise #2

Given the rectangle ABCD

AB=X the ratio between AB and BC is equal tox2 \sqrt{\frac{x}{2}}

We mark the length of the diagonal A A with m m

Check the correct argument:

XXXmmmAAABBBCCCDDD

Video Solution

Step-by-Step Solution

Let's find side BC

Based on what we're given:

ABBC=xBC=x2 \frac{AB}{BC}=\frac{x}{BC}=\sqrt{\frac{x}{2}}

xBC=x2 \frac{x}{BC}=\frac{\sqrt{x}}{\sqrt{2}}

2x=xBC \sqrt{2}x=\sqrt{x}BC

Let's divide by square root x:

2×xx=BC \frac{\sqrt{2}\times x}{\sqrt{x}}=BC

2×x×xx=BC \frac{\sqrt{2}\times\sqrt{x}\times\sqrt{x}}{\sqrt{x}}=BC

Let's reduce the numerator and denominator by square root x:

2x=BC \sqrt{2}\sqrt{x}=BC

We'll use the Pythagorean theorem to calculate the area of triangle ABC:

AB2+BC2=AC2 AB^2+BC^2=AC^2

Let's substitute what we're given:

x2+(2x)2=m2 x^2+(\sqrt{2}\sqrt{x})^2=m^2

x2+2x=m2 x^2+2x=m^2

Answer

x2+2x=m2 x^2+2x=m^2

Exercise #3

Given the rectangle ABCD

AB=X

The ratio between AB and BC is x2 \sqrt{\frac{x}{2}}

We mark the length of the diagonal A the rectangle in m

Check the correct argument:

XXXmmmAAABBBCCCDDD

Video Solution

Step-by-Step Solution

Given that:

ABBC=x2 \frac{AB}{BC}=\sqrt{\frac{x}{2}}

Given that AB equals X

We will substitute accordingly in the formula:

xBC=x2 \frac{x}{BC}=\frac{\sqrt{x}}{\sqrt{2}}

x2=BCx x\sqrt{2}=BC\sqrt{x}

x2x=BC \frac{x\sqrt{2}}{\sqrt{x}}=BC

x×x×2x=BC \frac{\sqrt{x}\times\sqrt{x}\times\sqrt{2}}{\sqrt{x}}=BC

x×2=BC \sqrt{x}\times\sqrt{2}=BC

Now let's focus on triangle ABC and use the Pythagorean theorem:

AB2+BC2=AC2 AB^2+BC^2=AC^2

Let's substitute the known values:

x2+(x×2)2=m2 x^2+(\sqrt{x}\times\sqrt{2})^2=m^2

x2+x×2=m2 x^2+x\times2=m^2

We'll add 1 to both sides:

x2+2x+1=m2+1 x^2+2x+1=m^2+1

(x+1)2=m2+1 (x+1)^2=m^2+1

Answer

m2+1=(x+1)2 m^2+1=(x+1)^2

Exercise #4

There are 18 balls in a box, 23 \frac{2}{3} of which are white.

How many white balls are there in the box?

Video Solution

Answer

12

Exercise #5

In a box there are 28 balls, 14 \frac{1}{4} of which are orange.

How many orange balls are there in the box?

Video Solution

Answer

7

Question 1

There are two circles.

The length of the radius of circle 1 is 6 cm.

The length of the diameter of circle 2 is 12 cm.

How many times greater is the area of circle 2 than the area of circle 1?

Question 2

There are two circles.

The length of the diameter of circle 1 is 4 cm.

The length of the diameter of circle 2 is 10 cm.

How many times larger is the area of circle 2 than the area of circle 1?

Question 3

How many times longer is the radius of the red circle than the radius of the blue circle?

220

Question 4

How many times longer is the radius of the red circle, which has a diameter of 24, than the radius of the blue circle, which has a diameter of 12?

Question 5

How many times longer is the radius of the red circle than the radius of the blue circle?

168

Exercise #6

There are two circles.

The length of the radius of circle 1 is 6 cm.

The length of the diameter of circle 2 is 12 cm.

How many times greater is the area of circle 2 than the area of circle 1?

Video Solution

Answer

They are equal.

Exercise #7

There are two circles.

The length of the diameter of circle 1 is 4 cm.

The length of the diameter of circle 2 is 10 cm.

How many times larger is the area of circle 2 than the area of circle 1?

Video Solution

Answer

614 6\frac{1}{4}

Exercise #8

How many times longer is the radius of the red circle than the radius of the blue circle?

220

Video Solution

Answer

5

Exercise #9

How many times longer is the radius of the red circle, which has a diameter of 24, than the radius of the blue circle, which has a diameter of 12?

Video Solution

Answer

2

Exercise #10

How many times longer is the radius of the red circle than the radius of the blue circle?

168

Video Solution

Answer

2 2

Question 1

How many times longer is the radius of the red circle than the radius of the blue circle?

210

Question 2

How many times longer is the radius of the red circle (14 cm) than the radius of the blue circle, which has a diameter of 7?

Question 3

ABCD is a deltoid with an area of 58 cm².

DB = 4

AE = 3

What is the ratio between the circles that have diameters formed by AE and and EC?

S=58S=58S=58333AAABBBCCCDDDEEE4

Exercise #11

How many times longer is the radius of the red circle than the radius of the blue circle?

210

Video Solution

Answer

212 2\frac{1}{2}

Exercise #12

How many times longer is the radius of the red circle (14 cm) than the radius of the blue circle, which has a diameter of 7?

Video Solution

Answer

4

Exercise #13

ABCD is a deltoid with an area of 58 cm².

DB = 4

AE = 3

What is the ratio between the circles that have diameters formed by AE and and EC?

S=58S=58S=58333AAABBBCCCDDDEEE4

Video Solution

Answer

3:26

Topics learned in later sections

  1. Scale Factors, Ratio and Proportional Reasoning
  2. Ratio
  3. Equivalent Ratios
  4. Direct Proportion
  5. Inverse Proportion