Relative Frequency in Probability

Relative Frequency in Probability

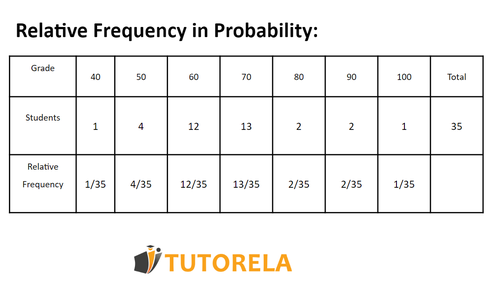
The relative frequency in probability represents the share of a certain object in the total number of objects in the set in question. In other words, Relative Frequency is a way of estimating probability based on past events. It is calculated by dividing the frequency of an event (how many times it happened) by the total number of trials or observations. For example, if you flip a coin 100 times and get 45 heads, the relative frequency of getting heads is 45100=0.45 \frac{45}{100} = 0.45. Relative frequency provides a practical approach to understanding probability, especially in experiments or real-life scenarios where actual outcomes can be measured, helping make predictions and decisions based on observed data.

Calculating Relative Frequency

Relative frequency is the ratio of the frequency of an event to the total number of trials. Calculated as:

Relative Frequency=Event FrequencyTotal Trials \text{Relative Frequency} = \frac{\text{Event Frequency}}{\text{Total Trials}}

Practical Use:

Relative frequency provides an empirical approach to probability by using observed data. For example, tracking how often it rains over many days helps predict future rain likelihood.

Let's demonstrate this with an example

There are 35 35 students in a university class.

At the end of the semester an exam was conducted and the results are shown in the following table:

We are asked to complete the last line of the table representing the relative frequency of each grade.

We do this by dividing the frequency of the grade (number of students who received that grade) by the total number of students (in our case 35 35 ).

For the grade 40 40 : the relative frequency is 135\frac{1}{35}

For the grade 50 50 : the relative frequency is 435\frac{4}{35}

For the score 60 60 : the relative frequency is 1235\frac{12}{35}

For the score 7070 : the relative frequency is 1335\frac{13}{35}

For the grade 80 80 : the relative frequency is 235\frac{2}{35}

For the grade 90 90 : the relative frequency is 235\frac{2}{35}

For the grade 100 100 : the relative frequency is 135\frac{1}{35}

A1 - Relative frequency in probability

If you are interested in this article you may also be interested in the following articles:

In Tutorela'sblog you will find a wide variety of mathematical articles.

Join Over 30,000 Students Excelling in Math!
Endless Practice, Expert Guidance - Elevate Your Math Skills Today
Related Subjects