The definition of the logarithm:
Change of Logarithm Base
Change of Logarithm Base
Reminder - Logarithms
Where:
is the base of the log
is what appears inside the log - can also appear inside of parentheses
is the exponent to which we raise the base of the log in order to obtain the number that appears inside of the log.
How to change the base of a logarithm?
According to the following rule:
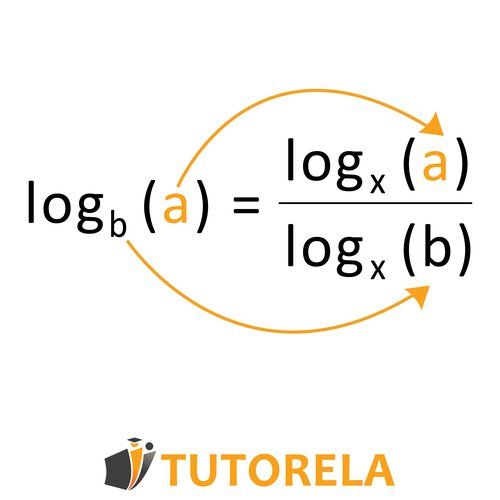
In the numerator there will be a log with the base we want to change to, as well as what appears inside of the original log.
In the denominator there will be a log with the base we want to change to, and the content will be the base of the original log.
Test yourself on change-of-base formula for logarithms!
\( \frac{\log_85}{\log_89}= \)
Change of Logarithm Base
Logarithm - Reminder
First, let's recall the definition of the log and understand what is the base of a logarithm:
The definition of the logarithm is:
Where:
is the base of the log
is what appears inside the log - which can also appear inside of parentheses
is the exponent to which we raise the base of the log to in order to obtain the number that appears inside of the log.
The rule states that if we raise base to the power of we obtain .
In order to solve a log, we ask ourselves - to what power should we raise to obtain .
The power that we found is the solution.
For example:
When we have the expression
Let's ask ourselves –
To what power do we need to raise to in order to obtain ? The answer is . Therefore:
Important to know - the log in the example is read as follows:
log base of
Change of logarithm base
You're probably asking yourself, why do we need to change the base of the logarithm?
Excellent question!
Sometimes in subtraction, addition, multiplication, or division exercises with different bases, it is easier for us to change the base of one of the logarithms so we can use the addition and subtraction formulas, and the same goes for multiplication and division.
So how do we do it? According to the following rule:
The Change of Base Rule for Logarithms:
Let's look at an example:
Here is the exercise:
Convert it to a log with base .
Solution:
Upon observation this is an illogical solution... To what power should we raise to in order to obtain ?
Therefore, we can change the base of the log and solve the problem far more easily! Note that the instruction is to change the log to base .
To change the base of the log, we'll use the formula:
In the numerator, we'll have log base (the base we want to change to) with the original log content
And in the denominator, we'll have log base (the base we want to change to) with the original base.
We obtain the following:
Are we done? Not yet. Now we must proceed to solve the logs until we obtain a number.
We insert the data into the exercise as seen below to obtain our solution:
Amazing!
Now we'll increase the difficulty level and move on to another exercise with a variable where we'll also use the change of logarithm base rule:
Solution
First of all, don't panic. With the rule you just learned, you can solve this exercise very simply. Let's start step by step.
When encountering two logarithms with different bases, the first step is to convert the larger log to the smaller one because it will be simpler to solve.
Let's do it - we'll convert to base and get:
Insert the data into the exercise as follows:
Let's continue solving the exercise.
We can solve the denominator -
Insert into the exercise
Let's continue solving the problem and write the exercise in a simpler form:
Let's combine like terms:
Solve the following –
Tip - Sometimes it's useful to substitute an auxiliary variable instead of the entire log.
When reached this stage in the exercise -
We can substitute
as follows:
But notice, this is not the result. Now we need to substitute instead of
and determine
we obtain the following solution :
\( \frac{\log_9e^2}{\log_9e}= \)
\( \log_74= \)
\( \frac{\log_89a}{\log_83a}= \)
Examples with solutions for Change-of-Base Formula for Logarithms
Exercise #1
Video Solution
Step-by-Step Solution
To solve this problem, let's simplify the given expression .
- Step 1: Recognize that both the numerator and denominator have the same base, 8.
- Step 2: The division property of logarithms states that .
- Step 3: Apply the division rule to the given expression: .
Thus, after simplifying, we see that .
Hence, the correct answer is , which corresponds to the choice 1.
Answer
Exercise #2
Video Solution
Step-by-Step Solution
To solve the given expression using the change-of-base formula, follow these steps:
- Step 1: Apply the change-of-base formula to both the numerator and the denominator expressions.
This gives us: and . - Step 2: Substitute these into our original expression:
. - Step 3: Simplify the fraction:
The cancels out from the numerator and the denominator, leaving us with . - Step 4: Further simplify using the fact that because any number to the power of 1 is .
This results in .
Therefore, the expression simplifies to .
The correct answer is , which matches choice 1.
Answer
Exercise #3
Video Solution
Step-by-Step Solution
To solve the problem of evaluating , we will use the change-of-base formula for logarithms.
The change-of-base formula is:
- , where can be any base, commonly chosen as 10 (common logs) or (natural logs).
We will choose natural logarithms () for simplicity, therefore:
By applying the change-of-base formula, we find that the logarithm can be expressed as .
Upon examining the provided choices, we identify that choice 2: matches our result.
Therefore, the solution to the problem is .
Answer
Exercise #4
Video Solution
Step-by-Step Solution
To solve this problem, we'll follow these steps:
- Step 1: Apply the mathematical property using the quotient rule for logarithms.
- Step 2: Simplify the expression into the desired format.
Now, let's work through each step:
Step 1: Given the expression , we can directly apply the quotient rule for logarithms, which tells us that .
Step 2: Applying this formula, we find that .
Therefore, the solution to the problem is .
Answer
Exercise #5
Video Solution
Step-by-Step Solution
To solve this problem, we'll simplify the given expression using logarithmic rules:
Step 1: Apply the power rule of logarithms:
The numerator can be rewritten using the power rule: .
Step 2: Substitute and simplify the fraction:
Substitute the expression from Step 1 into the original problem:
.
Step 3: Cancel common terms:
Since appears in both the numerator and the denominator, it cancels out, leaving:
.
Therefore, the solution to the problem is .
Answer
More Questions
Change-of-Base Formula for Logarithms
- Solve: Combined Logarithmic Fractions with Bases 7, 4, and 2
- Solve: log₈(x³)/log₈(x^1.5) + log₇(x⁵)/log₄₉(x) Logarithmic Expression
- Solve the Complex Logarithmic Equation: (log_x4 + log_x30.25)/(x*log_x11) + x = 3
- Solve the Complex Logarithm Equation: 1/log₂ₓ6 × log₂36 = log₅(x+5)/log₅2
- Solve the Complex Logarithmic Equation: (2ln4/ln5) + 1/log₅ = log₅(7x²+9x)









