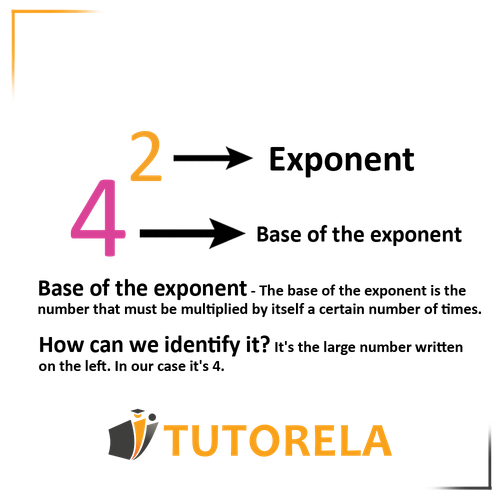
An exponent tells us the amount of times a number is to be multiplied by itself.
Powers and Roots Practice Problems - Basic Exponents
Master basic exponents and square roots with step-by-step practice problems. Learn how to solve powers like 4² and roots like √16 with guided exercises.
- Calculate basic exponents like 3², 5³, and identify base and exponent
- Solve square roots including √4, √16, and √25 using inverse operations
- Apply special exponent rules: any number to power 0 equals 1
- Recognize that any number to power 1 equals itself
- Practice mixed problems combining exponents and roots in expressions
- Build confidence with step-by-step solutions and detailed explanations
Understanding Powers and Roots - Basic
Exponents and Roots
What is an exponent?
What is a root?
A root is the inverse operation of exponentiation, which helps us discover which number multiplied by itself gives this result.
The square root is equal to the power of 0.5.
Practice Powers and Roots - Basic
\( \sqrt{25}= \)
Examples with solutions for Powers and Roots - Basic
Let's solve the problem step by step:
- Step 1: Understand what the square root means.
- Step 2: Apply this definition to the number .
- Step 3: Determine the correct number.
The square root of a number is a value that, when multiplied by itself, equals . This is written as .
We are looking for a number such that . This translates to finding .
We know that . Therefore, the principal square root of is .
Thus, the solution to the problem is .
Among the given choices, the correct one is: Choice 1: .
Answer:
6
The task is to find the square root of the number 100. The square root operation seeks a number which, when squared, equals the original number. For any positive integer, if , then should be our answer.
Step 1: Recognize that 100 is a perfect square. This means there exists an integer such that . Generally, we recall basic squares such as:
- and so forth, up to
Step 2: Checking integers, we find that:
Step 3: Confirm the result: Since , then .
Step 4: Compare with answer choices. Given that one of the choices is 10, and , choice 1 is correct.
Therefore, the square root of 100 is 10.
Answer:
10
To determine the square root of 16, follow these steps:
- Identify that we are looking for the square root of 16, which is a number that, when multiplied by itself, equals 16.
- Recall the basic property of perfect squares: .
- Thus, the square root of 16 is 4.
Hence, the solution to the problem is the principal square root, which is .
Answer:
4
To solve this problem, follow these steps:
- Step 1: Understand that the square root of a number is a value that, when multiplied by itself, equals .
- Step 2: Identify the number whose square is 81. Since , the square root of 81 is 9.
Therefore, the square root of 81 is .
Answer:
9
To solve this problem, we follow these steps:
- Step 1: Understand that finding the square root of a number means determining what number, when multiplied by itself, equals the original number.
- Step 2: Identify the numbers that could potentially be the square root of . These are , but by convention, the square root function typically refers to the non-negative root.
- Step 3: Calculate . This confirms that .
- Step 4: Verify using the problem's multiple-choice answers to ensure is among them, confirming choice number
.
Therefore, the solution to the problem is .
Answer:
7