Exponents and Roots - Basic

Exponentiation is the requirement for a number to be multiplied by itself several times.
In other words, when we see a number raised to a certain power, we know that we need to multiply the number by itself several times to reach the actual number.

Let's learn through an example:

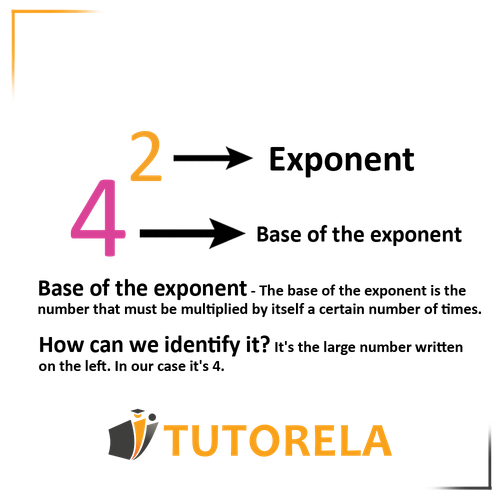
Exponent base – The exponent base is the number that is required to be multiplied by itself a certain number of times.
How do we identify it?
The main number written in large – in our example, this number is $4$.

Exponent – The exponent is the number that determines how many times the base is required to be multiplied by itself.
How do we identify it?
The exponent is the small number that appears to the right above the base – in our example, this number is $2$.

We read it like this: $4$ to the power of $2$.

To solve an exponent, we need to multiply the base of the exponent by itself the number of times the exponent requires us to.
Let's return to the example:

Base of the exponent = $4$
Exponent = $2$

Let's take the base of the exponent and multiply it by itself $2$ times.
We get:
$4*4=16$
and actually -
$4^2=16$

Let's practice another example.

How do you solve the following exponentiation:
$5^3=$

Solution:
Let's understand what the base and the exponent are.
Base of the exponent = $5$
Exponent = $3$
This means we need to multiply $5$ by itself $3$ times.
We get:
$5*5*5=125$
And actually:
$5^3=125$

Another example:

Solve the following power:
$3^3=$

Solution:
At first glance, we see that the base of the exponent and the exponent itself are identical. Does this change anything for us? Not at all, we work according to the rules.
We multiply the number $3$ by itself – for $3$ times and get:
$3*3*3=27$

And actually –
$3^3=27$

Another example:
Solve the following power:
$1^4=$
We need to take the number $1$ and multiply it by itself $4$ times. We get:
$1*1*1*1=1$
What would happen if we had such a power?
$1^{700}=$
Would we really need to write the number $1$ for $700$ times to understand that the result will be $1$ in the end?
No.
From this, we can conclude that: $1$ to the power of any number equals $1$.

Point to Ponder – What happens when the exponent is $1$?
When the exponent is $1$, the number does not change at all and it can be considered as if it has already performed the exponentiation.
For example:
$7^1=7$
Any number to the power of $1$ is the number itself.

Another point to consider – what happens when the exponent is $0$?
When the exponent of the number is $0$, we get a result of $1$. It doesn't matter what the number is.
Any number to the power of $0$ will equal $1$.
That means:
$2^0=1$
$​​​​​​​7^0=1$
${4,675}^0=1$

A root is equal to the power of $0.5$ and is denoted by the symbol $√$.
We can say that: $\sqrt{a}=a^{0.5}$
A root is the inverse operation of exponentiation.
If a small number appears on the left side, it will be the order of the root.

When any number appears as a regular root, we ask ourselves which number we would need to multiply by itself only twice to get the number inside the root?
In other words, which number raised to the power of $2$ will give us the number that appears inside the root.
For example:
$\sqrt4=2$

If we multiply $2$ by itself twice, we get $4$.

Another example:
$\sqrt16=$

Solution:
If we multiply the number $4$ twice by itself, we get $16$ and therefore:
$\sqrt16=4$

What should you know about roots?

• The result of the square root will always be positive!
  You will never get a negative result. We can get a result of $0$.
• There is no answer for $\sqrt{negative~number}$!

It is important to know - roots and exponents take precedence over all four arithmetic operations.
First, we perform the root and exponentiation operations, and only then do we proceed to the order of operations.