Sum of Squares
The sum of squares formula is one of the shortcut formulas, a key algebraic concept that helps express the sum of two squared terms:
That is, when we encounter two numbers with a plus sign (sum) and they are between parentheses and raised as an expression to the square, we can use this formula.
As mentioned, the formula is a shortcut, as it skips a step in the full solution:
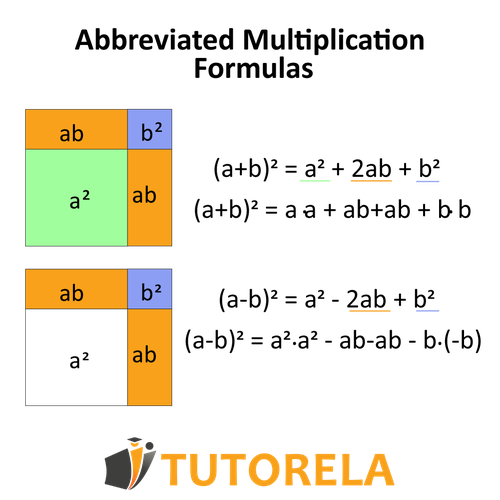
Pay attention - The formula also works for non-algebraic expressions or combined combinations with numbers and unknowns.
It's good to know that it is very similar to the formula for the difference of squares and differs only in the minus sign of the central element.
Example: