What is a square root?

What are those mysterious square roots that often confuse students and complicate their lives? The truth is that to understand them, we need to grasp the concept of the inverse operation.

What is a square root?

When we solve an exercise like $5=25^2$ , it's clear that $5$ times $5$ (that is, multiplying the number by itself) results in $25$ . This is the concept of a power, or to be more precise, a square power, which to apply, we must multiply the figure or the number by itself.

The concept of "square root" refers to the inverse operation of squaring numbers.

That is, if we have $X^2=25$ and we want to find the value of $X$ , what we need to do is perform an identical operation on both sides of the equation.

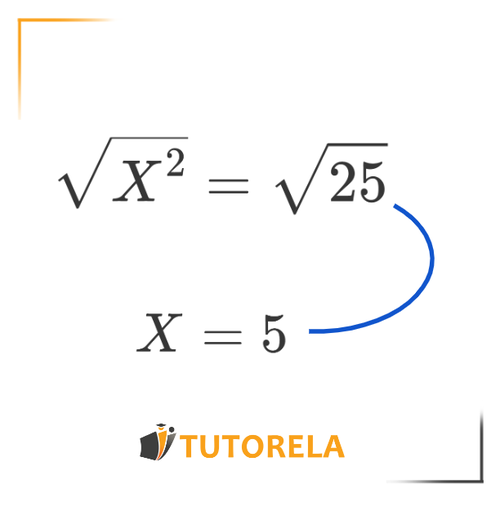
This operation is the square root.

So, we have: $\sqrt{X^2} = \sqrt{25}$ and the result is $X=5$ .

Detailed explanation

Start practice

Test yourself on square roots!

Choose the largest value

Practice more now

Now let's explain in more detail the operation we have performed.

On the left side of the equation, the square root cancels out the squared power (the square root and the squared power are inverse operations, remember?).
On the right side, we look for the figure or number that, when squared, gives us the result $25$ .

There are two numbers that meet these requirements: $5$ and $-5$ .

With that said, it's important to remember that the square root of a number will always be positive.

Therefore, to summarize, in the exercise: $X^2=25$ for example, we have two possible answers: $5$ and $-5$ .

If we are given the mathematical expression $\sqrt{25}$ the only possible answer will be $5$ .

Finding the Square Root Conditions

The only condition that must be met to find a square root is that the number under the square root must be positive.

You cannot find the square root of a negative number, that is, the expression $\sqrt{-25}$ is not correct and has no answer.

On the other hand,

the result of a square root does not necessarily have to be an integer,

that is, as long as the number under the square root is positive, we can find its square root.

For example: $\sqrt{89527}\approx 299.210$

Join Over 30,000 Students Excelling in Math!

Endless Practice, Expert Guidance - Elevate Your Math Skills Today

Test your knowledge

Question 1

$\sqrt{49}=$

Question 2

$\sqrt{36}=$

Question 3

$\sqrt{64}=$

Simple Square Root Calculation

Simple square roots are based on multiplication tables. Let's see some examples:

Example No. 1

$\sqrt{64}$

Solution: The square root, as we have already seen, is the inverse operation of squaring a number. Therefore, we must ask ourselves which number squared, or which number multiplied by itself, will result in $64$ .

Given that: $8^2=64$

the answer will be: $\sqrt{64} = 8$

Do you know what the answer is?

Question 1

Solve the following exercise:

$\sqrt{x^2}=$

Question 2

$\sqrt{441}=$

Question 3

$\sqrt{x}=1$

$$ X=? $$

Example No. 2

$\sqrt{49}$

Solution: in this case, we also have to ask ourselves what number squared, or what number multiplied by itself, results in $49$ .

Given that: $7^2=49$ the answer will be: $\sqrt{49} = 7$

Example No. 3

$\sqrt{9}$

Solution: following the same logic, we see that $3^2=9$ and, therefore, the answer will be: $\sqrt{9} = 3$ .

Check your understanding

Question 1

$\sqrt{x}=2$

Question 2

$\sqrt{x}=6$

Question 3

$\sqrt{4}=$

Mathematical Operations and Square Roots

In this section, we will apply what we have learned so far regarding square roots and see how we can use this information to solve algebraic exercises that include square roots.

An important rule that we must remember when solving these types of exercises is that:

Square roots should be solved first, that is, before any other mathematical operation that is outside of the square root itself.

Exercises with Solutions

Exercise No. 1

$10 + \sqrt{81} =$

Solution:

First, we will solve the square root, as it comes before the addition that is outside of it.

Thus, we obtain:

$\sqrt{81} = 9$

Then we continue with the rest of the exercise:

$10+9=19$

Therefore, the result is $10 + \sqrt{81} = 19$

Do you think you will be able to solve it?

Question 1

$\sqrt{9}=$

Question 2

$\sqrt{16}=$

Question 3

$\sqrt{36}=$

Exercise No. 2

$3 \times \sqrt{16} +8=$

Solution:

This exercise is a bit more complicated. Initially, we must solve the square root, as this operation precedes any other in the exercise.

Thus, we obtain: $\sqrt{16} = 4$

Afterwards, we should approach the exercise like any other math problem: $3 \times 4 +8$ .

According to the order of operations in math, multiplication and division come before addition and subtraction. Therefore, the result is: $20$ .

The answer to the exercise is: $3 \times \sqrt{16} +8=20$

Exercise No. 3

$\sqrt{36} \div3+\sqrt{81}\times2=$

Solution:

Here we also need to solve the square roots first.

$\sqrt{36}=6$

$\sqrt{81}=9$

We insert the numbers and solve according to the order of operations:

$6\div3+9\times2=2+18=20$

Therefore, the answer is:

$\sqrt{36} \div3+\sqrt{81}\times2=20$

Examples and Exercises with Solutions for Square Roots

Exercise #1

Choose the largest value

Video Solution

Step-by-Step Solution

Let's begin by calculating the numerical value of each of the roots in the given options:

$\sqrt{25}=5\\ \sqrt{16}=4\\ \sqrt{9}=3\\$ We can determine that:

$5>4>3>1$ Therefore, the correct answer is option A

Answer

$\sqrt{25}$

Exercise #2

$\sqrt{49}=$

Video Solution

Step-by-Step Solution

To solve this problem, we follow these steps:

Step 1: Understand that finding the square root of a number means determining what number, when multiplied by itself, equals the original number.
Step 2: Identify the numbers that could potentially be the square root of $49$ . These are $\pm7$ , but by convention, the square root function typically refers to the non-negative root.
Step 3: Calculate $7 \times 7 = 49$ . This confirms that $\sqrt{49} = 7$ .
Step 4: Verify using the problem's multiple-choice answers to ensure $7$ is among them, confirming choice number .

Therefore, the solution to the problem $\sqrt{49}$ is $7$ .

Answer

Exercise #3

$\sqrt{36}=$

Video Solution

Step-by-Step Solution

Let's solve the problem step by step:

Step 1: Understand what the square root means.

The square root of a number $n$ is a value that, when multiplied by itself, equals $n$ . This is written as $x = \sqrt{n}$ .

Step 2: Apply this definition to the number $36$ .

We are looking for a number $x$ such that $x^2 = 36$ . This translates to finding $x = \sqrt{36}$ .

Step 3: Determine the correct number.

We know that $6 \times 6 = 36$ . Therefore, the principal square root of $36$ is $6$ .

Thus, the solution to the problem is $\sqrt{36} = 6$ .

Among the given choices, the correct one is: Choice 1: $6$ .

Answer

Exercise #4

$\sqrt{64}=$

Video Solution

Step-by-Step Solution

To solve this problem, we'll follow these steps:

Step 1: Recognize what finding a square root means
Step 2: List known perfect squares to identify which one results in 64
Step 3: Verify the square root by calculation

Now, let's work through each step:
Step 1: To find the square root of 64, we seek a number that, when multiplied by itself, equals 64.
Step 2: Consider the sequence of perfect squares: $1^2 = 1$ , $2^2 = 4$ , $3^2 = 9$ , $4^2 = 16$ , $5^2 = 25$ , $6^2 = 36$ , $7^2 = 49$ , $8^2 = 64$ .
Step 3: We see that $8^2 = 64$ . Therefore, the square root of 64 is 8.

Therefore, the solution to this problem is $8$ .

Answer

Exercise #5

Solve the following exercise:

$\sqrt{x^2}=$

Video Solution

Step-by-Step Solution

In order to simplify the given expression, we will use two laws of exponents:

a. The definition of root as an exponent:

$\sqrt[n]{a}=a^{\frac{1}{n}}$ b. The law of exponents for power of a power:

$(a^m)^n=a^{m\cdot n}$

Let's start with converting the square root to an exponent using the law mentioned in a':

$\sqrt{x^2}= \\ \downarrow\\ (x^2)^{\frac{1}{2}}=$ We'll continue using the law of exponents mentioned in b' and perform the exponent operation on the term in parentheses:

$(x^2)^{\frac{1}{2}}= \\ x^{2\cdot\frac{1}{2}}\\ x^1=\\ \boxed{x}$ Therefore, the correct answer is answer a'.

Answer

$x$

Test your knowledge

Question 1

$\sqrt{64}=$

Question 2

$\sqrt{100}=$

Question 3

Choose the largest value

Start practice

What is a square root?

What is a square root?

This operation is the square root.

Test yourself on square roots!

Finding the Square Root Conditions

Simple Square Root Calculation

Example No. 1

Example No. 2

Example No. 3

Mathematical Operations and Square Roots

Exercises with Solutions

Exercise No. 1

Exercise No. 2

Exercise No. 3

Examples and Exercises with Solutions for Square Roots

Exercise #1

Video Solution

Step-by-Step Solution

Answer

Exercise #2

Video Solution

Step-by-Step Solution

Answer

Exercise #3

Video Solution

Step-by-Step Solution

Answer

Exercise #4

Video Solution

Step-by-Step Solution

Answer

Exercise #5

Video Solution

Step-by-Step Solution

Answer

More Questions

Square Roots