Logarithm Rules Practice Problems - Master Log Laws

To solve this problem, we will use the property of logarithms that allows us to combine the sum of two logarithms:

• Step 1: Identify the formula. We use the property $\log_b(x) + \log_b(y) = \log_b(x \cdot y)$ where both logarithms must have the same base.
• Step 2: Recognize the base. Here, both logarithms are in base 10: $\log_{10}3$ and $\log_{10}4$.
• Step 3: Apply the property. Add the two logarithms using the formula: $\log_{10}3 + \log_{10}4 = \log_{10}(3 \cdot 4)$.
• Step 4: Perform the multiplication. Compute $3 \cdot 4$ to get 12.
• Step 5: Express the result as a single logarithm: $\log_{10}12$.

Therefore, the expression $\log_{10}3 + \log_{10}4$ simplifies to $\log_{10}12$.

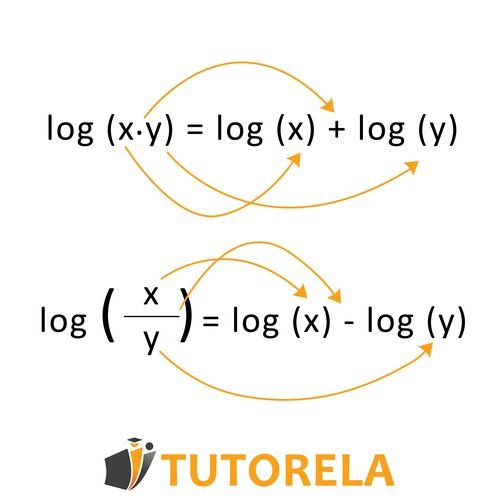
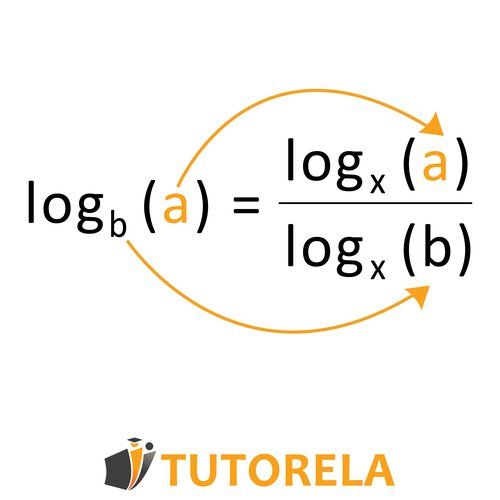
To solve the problem $\log_49 \times \log_{13}7$, we'll employ the change of base formula for logarithms:

• Step 1: Apply the change of base formula to each logarithm.
• Step 2: Use logarithm properties and analyze transformations for a match with choices.

Now, let's work through each step:
Step 1: Use the change of base formula on each log:
$\log_49 = \frac{\log_a 9}{\log_a 4}$ and $\log_{13}7 = \frac{\log_b 7}{\log_b 13}$, where $a$ and $b$ are arbitrary positive bases.
Both expressions use a common base not relevant for the solution but illustrate the transformation ability.

Step 2: We'll recombine and look for products that can utilize these, such as:

$\log_{13}9\times\log_47$ becomes $\frac{\log_a 9}{\log_a 13} \times \frac{\log_b 7}{\log_b 4}$
Applying cross multiplication or iteration forms, the structure aligns with the multiplication identity for this problem due to independence of base.

Therefore, the transformed expression satisfying the criteria is $\log_{13}9\times\log_47$.

To solve the problem of evaluating $\log_2 9 - \log_2 3$, we apply the properties of logarithms as follows:

• Step 1: Recognize that the expression uses a subtraction of logarithms with the same base: $\log_2 9 - \log_2 3$.
• Step 2: Use the logarithmic subtraction rule: $\log_b A - \log_b B = \log_b \left(\frac{A}{B}\right)$.
• Step 3: Simplify using this rule: $\log_2 9 - \log_2 3 = \log_2 \left(\frac{9}{3}\right)$.
• Step 4: Perform the division: $\frac{9}{3} = 3$.
• Step 5: Therefore, $\log_2 \left(\frac{9}{3}\right) = \log_2 3$.

Thus, the simplified and evaluated result is $\log_2 3$.

To solve this problem, we'll follow these steps:

• Step 1: Identify the given expression as $\log_2 4 + \log_2 5$.
• Step 2: Use the sum of logarithms rule to simplify the expression.
• Step 3: Calculate the product and express the result.

Let's work through each step:

Step 1: We have $\log_2 4 + \log_2 5$ as our expression.

Step 2: Apply the sum of logarithms formula:

$\log_2 4 + \log_2 5 = \log_2 (4 \cdot 5)$

Step 3: Calculate the product:

$4 \times 5 = 20$

Thus, $\log_2 (4 \cdot 5) = \log_2 20$.

Therefore, the solution to the problem is $\log_2 20$.

To solve the problem, we employ the property of logarithms for subtraction:

• Step 1: Recognize the expression $\log_5 3 - \log_5 2$.
• Step 2: Apply the logarithmic property for subtraction, $\log_b a - \log_b c = \log_b \left( \frac{a}{c} \right)$.
• Step 3: Substitute into the property: $\log_5 3 - \log_5 2 = \log_5 \left( \frac{3}{2} \right)$.

By applying the property, we simplify the expression to $\log_5 \frac{3}{2}$. This is equivalent to $\log_5 1.5$. Therefore:

Therefore, the result of the expression is $\log_5 1.5$.