The similarity ratio is the constant difference between the corresponding sides of the two shapes.
That is, if the similarity ratio is , we know that each side of the large triangle is times larger than that of the small triangle.
Similarity ratio
What is the similarity ratio?
How do we calculate the similarity ratio?
The calculation of the similarity ratio is divided into several steps that must be performed:
- First we must know that we are dealing with similar triangles or polygons.
- We must know how to identify the corresponding sides in each of the triangles or polygons.
- We need to know the sizes of a pair of equal sides.
- We must divide the size of one side by the size of the other side.
The result obtained is actually the similarity ratio.
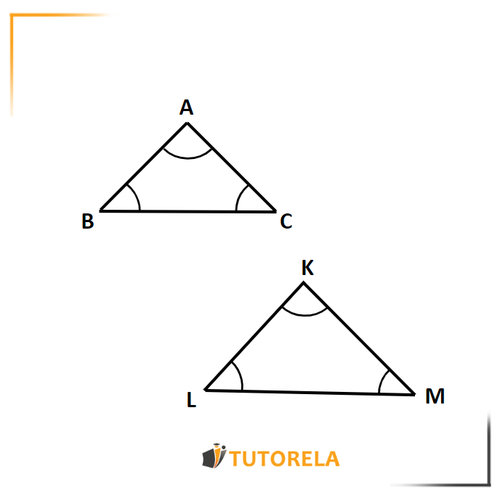
Test yourself on the ratio of similarity!
Is the similarity ratio between the three triangles equal to one?
We will exemplify this through an exercise.
In the drawing before us there are two triangles similar to and .
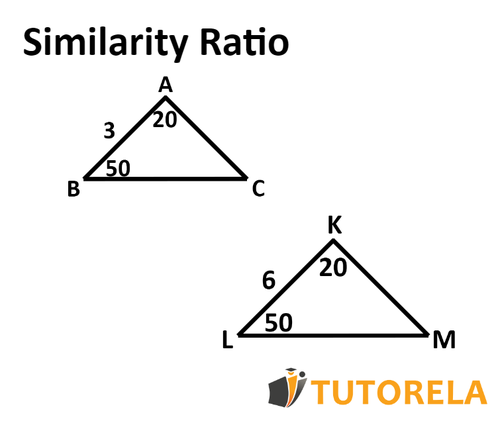
We are required to calculate the similarity ratio between the two triangles.
We are going to work according to the steps described above.
The first step is actually completed - It has been given to us, because these are two similar triangles.
In the second step, we must identify the corresponding sides in each of the two triangles. We will look at the drawing and see that the two triangles have angles. The angle is equal and equal to the angle and the angle is equal to the angle .
From this we can conclude that, in terms of location, the sides and are corresponding sides.
The third step is fairly easy, because we are given the sizes of these two sides, ,
In the fourth and final step, we will perform a simple operation of dividing the sizes of the corresponding sides.
We obtain:
We obtain that the similarity ratio of these two similar triangles is equal to .
If you are interested in this article you may also be interested in the following articles
- Similarity of Triangles and Polygons
- Similar Triangles
- Criterion of similarity between two triangles
- Similarity of geometric figures
In Tutorela you will find a great variety of mathematics articles.
Similarity ratio exercises
Exercise 1
Task
Given:
Choose the correct answer
Solution
According to the two triangles are similar.
Answer
Answers
Given that triangles ABC and DEF are similar, what is their ratio of similarity?
BC is parallel to DE.
Fill in the gap:
\( \frac{AD}{}=\frac{AE}{AC} \)
According to which theorem are the triangles similar?
What is their ratio of similarity?
Exercise 2
Task
Similar triangles:
Solution
The triangles are similar as a result of the similarity ratio.
Answer
Exercise 3
Question
What is the ratio between the sides of the triangles and ?
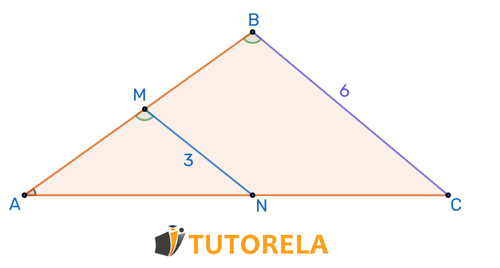
Solution
From image comes out:
Answer
Exercise 4:
Task
Choose the correct answer
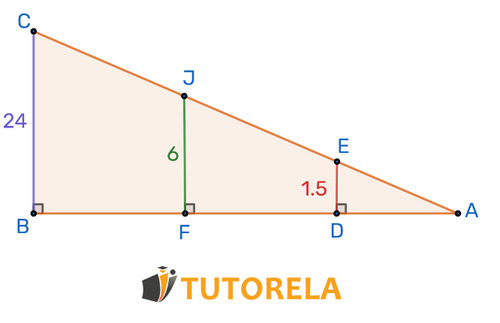
Solution
Ratio of similarity
Answer
According to which theorem are the triangles congruent in the diagram?
Complete the similarity ratio:
\( \frac{AB}{DF}=\frac{BC}{}=\frac{}{EF} \)
Triangle DFE is similar to triangle ABC.
Calculate the length of FE.
Complete the similarity ratio given that the triangles below are similar:
\( \frac{AB}{}=\frac{}{EF}=\frac{AC}{} \)
Exercise 5
Question
Find the similarity ratio corresponding to the triangles and .
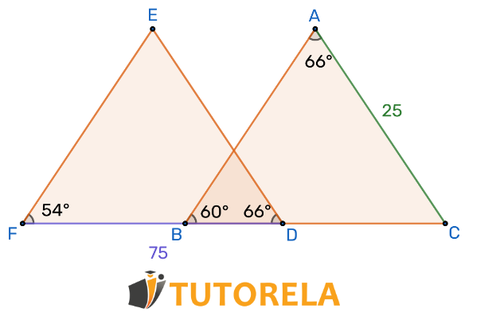
Solution
It follows that
Answer
Review questions
What is the similarity ratio?
It is the ratio of the corresponding sides of two similar figures.
How to get the similarity ratio?
The similarity ratio is obtained by dividing the corresponding sides of two similar figures. Let's see an example:
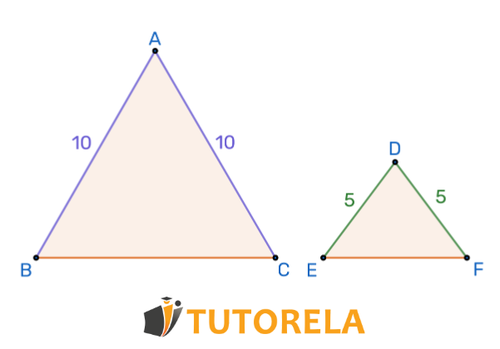
Given the following similar triangles.
Calculate the similarity ratio

Given that
Then we must locate which are the corresponding sides, and from this we deduce that
Then the corresponding sides are ,
Now to calculate the similarity ratio we do the quotient of these two sides.
Therefore the similarity ratio is
What are two similar triangles?
We can say that two triangles are similar when they have the same shape even if they have different sizes, for that they must meet some of the following similarity criteria:
- Side-Side-Side (SSS): If the ratio of their three pairs of corresponding sides is the same then two triangles are similar.
- Side-Angle-Side (SAS): Two triangles are similar if the ratio of two pairs of corresponding sides is the same and the angle between these two pairs is the same, then they are similar triangles.
- Angle-Angle (AA): For two triangles to be similar by this criterion, two of their respective angles must measure the same and therefore the third angle must also have the same measure as the angle corresponding to that angle. That is, their three corresponding angles measure the same.
What are congruent figures?
Unlike similar figures that do not necessarily have to equal side lengths, two figures are congruent when they have the same shape AND their corresponding sides are equal lengths.
What is the similarity ratio of two rectangles?
Just like similar triangles, to calculate the similarity ratio we must calculate the quotient of the corresponding sides. Let's see an example:
Given the following similar rectangles
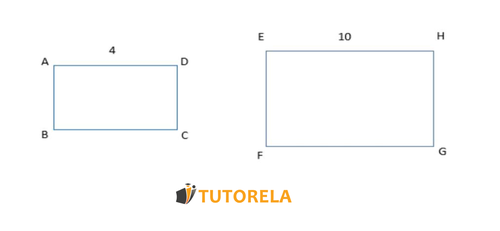
Find the similarity ratio
Since they are similar rectangles and because they are quadrilaterals they have right angles, then we can deduce their corresponding sides:
One of their corresponding sides are ,, then we can calculate the similarity ratio.
Therefore the similarity ratio is
\( ΔACB∼ΔBED \)
Choose the correct answer.
What is the ratio of similarity between the triangles shown in the diagram below?
What is the scale factor between the two triangles below?
Examples with solutions for The Ratio of Similarity
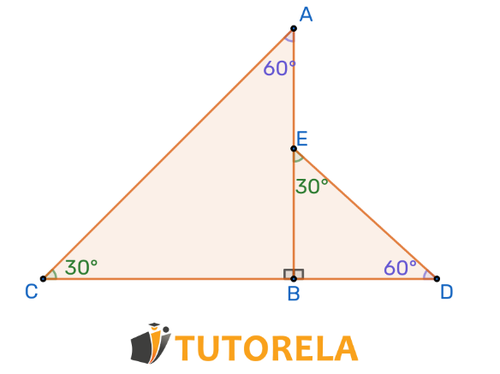
Exercise #1
Choose the correct answer.
Video Solution
Step-by-Step Solution
First, let's look at angles C and E, which are equal to 30 degrees.
Angle C is opposite side AB and angle E is opposite side BD.
Now let's look at angle B, which is equal to 90 degrees in both triangles.
In triangle ABC the opposite side is AC and in triangle EBD the opposite side is ED.
Let's look at angles A and D, which are equal to 60 degrees.
Angle A is the opposite side of CB, angle D is the opposite side of EB
Therefore, from this it can be deduced that:
And also:
Answer
Answers a + b are correct.
Exercise #2
What is the ratio between the sides of the triangles ΔABC and ΔMNA?
Video Solution
Step-by-Step Solution
From the data in the drawing, it seems that angle M is equal to angle B
Also, angle A is an angle shared by both triangles ABC and AMN
That is, triangles ABC and AMN are similar respectively according to the angle-angle theorem.
According to the letters, the sides that are equal to each other are:
Now we can calculate the ratio between the sides of the given triangles:
Answer
Exercise #3
What is the ratio of similarity between the triangles shown in the diagram below?
Video Solution
Step-by-Step Solution
From the drawing it appears that angle E equals angle A
Since angle D equals 90 degrees, its adjacent angle also equals 90 degrees.
In other words, angle D1 equals angle D2 and both equal 90 degrees.
Since we have two pairs of equal angles, the triangles are similar.
Also angle B equals angle C
Now let's write the similar triangles according to their corresponding angle letters:
Let's write the ratio of sides according to the corresponding letters of the similar triangles:
Answer
Exercise #4
Triangle DFE is similar to triangle ABC.
Calculate the length of FE.
Video Solution
Step-by-Step Solution
Let's look at the order of letters of the triangles that match each other and see the ratio of the sides.
We will write accordingly:
Triangle ABC is similar to triangle DFE
The order of similarity ratio will be:
Now let's insert the existing data we have in the diagram:
Let's reduce y and we get:
Answer
Exercise #5
BC is parallel to DE.
Fill in the gap:
Video Solution
Step-by-Step Solution
Since we are given that line BC is parallel to DE
Angle E equals angle C and angle D equals angle B - corresponding angles between parallel lines are equal.
Now let's observe that angle D is opposite to side AE and angle B is opposite to side AC, meaning:
Now let's observe that angle E is opposite to side AD and angle C is opposite to side AB, meaning:
Answer
AB
More Questions
The Ratio of Similarity
- Similar Triangles: Finding Perimeter of ΔDEF When ΔABC Perimeter is 64
- Similar Triangles: Calculate Perimeter Using 12-13-5 Triangle Measurements
- Similar Triangles: Identify Matching Pairs and Calculate Their Ratio
- Triangle Height Problem: Finding Perpendicular Distance of 24 Units
- Similar Triangles Analysis: Finding Similarity Ratio Among Three Given Triangles
- Congruent Triangles
- Congruence Criterion: Side, Angle, Side
- Congruence Criterion: Angle, Side, Angle
- Congruence Criterion: Side, Side, Side
- Side, Side, Angle
- Congruent Rectangles
- Similarity of Triangles and Polygons
- Similarity of Geometric Figures
- Similar Triangles
- Triangle similarity criteria
- Sum of Angles in a Polygon
- Sum of the Interior Angles of a Polygon
- Exterior angles of a triangle
- Sum of the Exterior Angles of a Polygon
- Relationships Between Angles and Sides of the Triangle
- Relations Between The Sides of a Triangle









