Order of Operations Practice Problems: PEMDAS & BODMAS

Master the order of operations with step-by-step practice problems covering addition, subtraction, multiplication, and division using PEMDAS/BODMAS rules.

📚Practice Order of Operations with Interactive Problems

Apply PEMDAS/BODMAS rules to solve multi-step arithmetic problems correctly
Practice multiplication and division before addition and subtraction
Solve combined operations from left to right systematically
Master the correct sequence: parentheses, powers, multiplication/division, addition/subtraction
Build confidence with step-by-step worked examples and solutions
Avoid common mistakes in order of operations calculations

Understanding Addition, Subtraction, Multiplication and Division

Complete explanation with examples

The Correct Order when Solving Combined Operations

The rules for the order of operations in an addition and subtraction exercise are quite simple.

In exercises with combined operations in which there are also multiplications and divisions, the order of operations is as follows:

Parentheses
Powers and roots
Multiplications and divisions
Addition and subtraction

Illustration of BODMAS/PEMDAS rule hierarchy for arithmetic operations: Brackets/Parentheses, Order/Exponents, Division/Multiplication, Addition/Subtraction explained for basic mathematical calculations.

Detailed explanation

Practice Addition, Subtraction, Multiplication and Division

Test your knowledge with 23 quizzes

$$ 21-3-6+9-5= $$

Examples with solutions for Addition, Subtraction, Multiplication and Division

Step-by-step solutions included

Exercise #1

$100+5-100+5$

Step-by-Step Solution

$100+5-100+5=105-100+5=5+5=10$

Answer:

Video Solution

Exercise #2

$3+2-1=$

Step-by-Step Solution

According to the rules of the order of operations, given that the exercise only involves subtraction and addition operations, we solve the exercise from left to right:

$3+2=5$

$5-1=4$

Answer:

$4$

Video Solution

Exercise #3

Solve:

$9-3+4-2$

Step-by-Step Solution

According to the rules of the order of operations, we will solve the exercise from left to right since it only has addition and subtraction operations:

$9-3=6$

$6+4=10$

$10-2=8$

Answer:

Video Solution

Exercise #4

$9+3-1=$

Step-by-Step Solution

According to the rules of the order of arithmetic operations, we will work to solve the exercise from left to right:

9+3=11

11-1=10

And this is the solution!

Answer:

$11$

Video Solution

Exercise #5

$-7+5+2+1=$

Step-by-Step Solution

According to the rules of the order of arithmetic operations, we solve the exercise from left to right since it only has addition and subtraction operations:

$-7+5=-2$

$-2+2=0$

$0+1=1$

Answer:

$1$

Video Solution

Frequently Asked Questions

Everything you need to know about Addition, Subtraction, Multiplication and Division

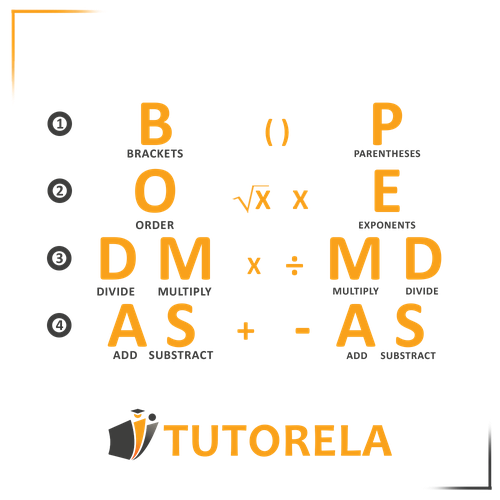
What is the correct order of operations in math?

The order of operations follows PEMDAS/BODMAS: Parentheses/Brackets first, then Exponents/Orders (powers and roots), then Multiplication and Division from left to right, and finally Addition and Subtraction from left to right.

Do you multiply before adding in order of operations?

Yes, multiplication is always performed before addition according to the order of operations. This is why 2 + 3 × 4 equals 14 (not 20), because you first calculate 3 × 4 = 12, then add 2.

How do you solve order of operations problems step by step?

Follow these steps: 1) Solve anything in parentheses first, 2) Calculate powers and roots, 3) Perform multiplication and division from left to right, 4) Finally, do addition and subtraction from left to right.

What are common mistakes students make with order of operations?

Common mistakes include: working from left to right without following PEMDAS, adding before multiplying, forgetting to solve parentheses first, and not working multiplication/division or addition/subtraction from left to right when they appear together.

Why is order of operations important in math?

Order of operations ensures everyone gets the same answer to mathematical expressions. Without these rules, expressions like 2 + 3 × 4 could be interpreted differently, leading to confusion and incorrect calculations in advanced math.

How do you remember the order of operations?

Use memory devices like PEMDAS (Please Excuse My Dear Aunt Sally) or BODMAS (Brackets, Orders, Division/Multiplication, Addition/Subtraction). Both represent the same sequence of operations.

What does left to right mean in order of operations?

When operations have equal priority (like multiplication and division, or addition and subtraction), you solve them in order from left to right. For example, in 20 ÷ 4 × 2, you first do 20 ÷ 4 = 5, then 5 × 2 = 10.

Can you give an example of order of operations with all four basic operations?

Example: 100 ÷ 2 - 10 ÷ 2 + 3 × 4 - 6. First do multiplication/division: 50 - 5 + 12 - 6. Then addition/subtraction from left to right: 45 + 12 - 6 = 57 - 6 = 51.

More Addition, Subtraction, Multiplication and Division Questions

Click on any question to see the complete solution with step-by-step explanations

Addition, Subtraction, Multiplication and Division

Continue Your Math Journey

Master Addition, Subtraction, Multiplication and Division first, then advance to these related topics that build on your fraction skills

Topics Learned in Later Sections

Order of Operations: Exponents Order of Operations: Roots Order of Operations - Exponents and Roots Order of Operations with Parentheses Division and Fraction Bars (Vinculum)The Numbers 0 and 1 in Operations Neutral Element (Identity Element)Order or Hierarchy of Operations with Fractions Multiplicative Inverse Special cases (0 and 1, reciprocals, fraction line)The Order of Operations

Practice by Question Type

Choose your preferred learning style and practice format for fraction problems

Addition, subtraction, multiplication and division Complete the missing numbers Decimal numbers Identify the greater value Only addition and subtraction Solving the problem Using fractions Using parentheses Worded problems