Methods for solving a quadratic function

In this article, we will learn the three most common ways to solve a quadratic function easily and quickly.

Trinomial
Quadratic Formula
Completing the Square

Reminder:

The basic quadratic function equation is:
$Y=ax^2+bx+c$

When:
$a$ - the coefficient of $X^2$
$b$ - the coefficient of $X$
$c$ - the constant term

$a$ must be different from $0$
$b$ or $c$ can be $0$
$a,b,c$ can be negative/positive
The quadratic function can also look like this:
- $Y=ax^2$
- $Y=ax^2+bx$
- $Y=ax^2+c$

Detailed explanation

Start practice

Test yourself on solving quadratic equations!

a = coefficient of x²

b = coefficient of x

c = coefficient of the independent number

$$ 5-6x^2+12x=0 $$

What are the components of the equation?

Practice more now

How do you solve a quadratic function using trinomial?

$Y=ax^2+bx+c$
Find $2$ numbers that satisfy the following two conditions:

$first~number \cdot second~number = c \cdot a$
$first~number + second~number = b$

How do we proceed?
First of all, let's write down on the side:

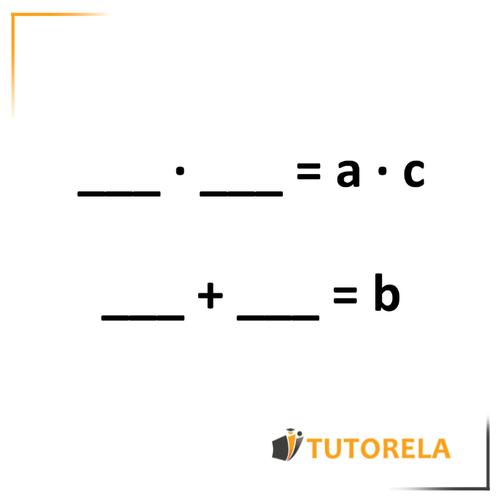
A1 - Cómo se resuelve la función cuadrática mediante un trinomio

Then–

We will find all the numbers whose product is $a\cdot c$ and write them down.
We will check which pair of numbers from the pairs we found earlier will give us the sum $b$ .
We will write the pair of numbers that meets the $2$ conditions in this form:
$0= (x+one~solution)(x+second~solution)$
or with subtraction operations.
$2$ solutions of the quadratic equation will be those that solve the equation above (we will reverse the sign for the pair of numbers we found)

Tip – It is recommended to use the trinomial method when $a=1$

For more reading and practice on trinomials, click here!

And now let's practice!
Solve the quadratic function in front of you using trinomial:
$x^2-9x+14$

Solution:
First of all, let's write down on the side:

Resuelve la siguiente función cuadrática mediante un trinomio

Let's find all the numbers whose product is $14$ (and remember the negative numbers as well)
We get:
$14,1$
$7,2$
$-14, -1$
$-7 ,-2$

Now, let's check which pair of numbers from the pairs we found earlier will give us the sum (-9)

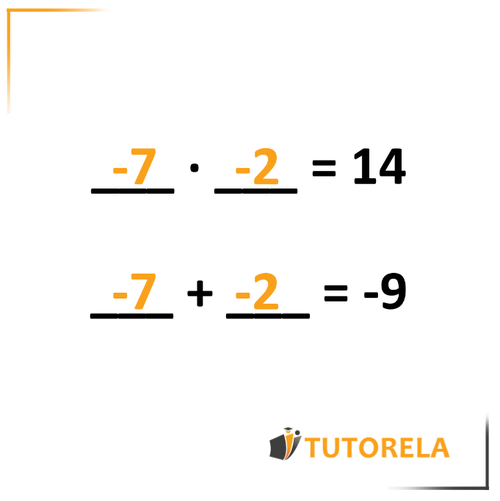
Hallemos todos los números cuyo producto sea 14

The pair of numbers that managed to meet both conditions is $-7 ,-2$

We write the factorization:
$(x-7)(x-2)=0$
The solutions:
$X=7$
$X=2$

How do you solve a quadratic function using the quadratic formula?

$Y=ax^2+bx+c$

Meet the quadratic formula:
$x = {-b \pm \sqrt{b^2-4ac} \over 2a}$

All you need to do is arrange the parameters of the quadratic function, substitute into the equation once with a plus and once with a minus, and find the solutions.

To learn more about the quadratic formula, click here!

Let's practice!
In front of us is the quadratic function:
$2x^2-3x+1$

Let's solve it using the quadratic formula:
First, let's arrange the parameters:
$a=2$
$b=-3$
$c=1$

Now, we substitute into the quadratic formula:
For the first time with plus-

$\frac{-(-3)+\sqrt{(-3)^2-4*2*1}}{2*2}=$

$=\frac{3+\sqrt1}4=\frac{4}4=1$

The second time with minus:

$\frac{-(-3)-\sqrt{(-3)^2-4*2*1}}{2*2}=$

$=\frac{3-\sqrt1}4=\frac{2}4=\frac{1}2$

We got $2$ solutions –
$X=\frac{1}2,1$

Join Over 30,000 Students Excelling in Math!

Endless Practice, Expert Guidance - Elevate Your Math Skills Today

Test your knowledge

Question 1

a = coefficient of x²

b = coefficient of x

c = coefficient of the independent number

$$ 10x^2+5+20x=0 $$

What are the components of the equation?

Question 2

a = coefficient of x²

b = coefficient of x

c = coefficient of the independent number

$$ -8x^2-5x+9=0 $$

What are the components of the equation?

Question 3

a = coefficient of x²

b = coefficient of x

c = coefficient of the independent number

$$ -x^2-2=0 $$

What are the components of the equation?

How do you solve a quadratic function by completing the square?

To use the method of completing the square, let's recall some of the formulas for the shortened multiplication:

$a^2+2ab+b^2=(a+b)^2$

$a^2-2ab+b^2=(a-b)^2$

Solution method with example:
Here is the function $4x^2+8x-5$

Let's look at the quadratic function and focus only on $ax^2+bx$ .
For now, we'll ignore $c$ . In the example, we'll focus on $4x^2+8x$
Let's recall the formulas for completing the square and ask, what expression can we put inside the parentheses squared, that is, which $(a-b)^2$ or $(a+b)^2$ as relevant, that will give us what appears in the pair we focused on $ax^2+bx$

In the example-
The appropriate formula for completing the square is:
$a^2+2ab+b^2=(a+b)^2$

Let's ask, what should we put as $a$ and $b$ to get $4x^2+8x$ ?
The answer is - $(2x+2)^2$
Let's expand this expression according to the formula for completing the square and get:
$4x^2+8x+4$

Note that the expression in parentheses also brings with it a certain number and not just the pair we focused on, so we will need to cancel it.
If the added number is negative, we will add it to the equation to cancel it, and if the number is positive, we will subtract it from the equation to cancel it.
Additionally, we will return to $C$ from the original function and write it in the equation as well.

In the example:
$4x^2+8x+4$
The number $4$ was added. To cancel it, we will subtract $4$ and not forget the original $C$
$4x^2+8x-4-5=$
We will substitute the pair $ax^2+bx$ with the appropriate expression in parentheses squared that we found and arrange the equation – we will get the completion of the square.

In the example:
$(2X+2)^2-4-5=$
$(2x+2)^2-9$

Now let's set the equation to 0 and solve:
$(2x+2)^2-9=0$

$(2x+2)^2=9$

$(2x+2)^2=3^2$
and also
$(2x+2)^2=(-3)^2$

Solution 1:
$2x+2=3$
$2x=1$
$x=\frac{1}2$

Solution 2:
$2x+2=-3$
$2x=-5$
$x=-2\frac{1}2$

For more information on completing the square, click here!

Do you know what the answer is?

Question 1

a = coefficient of x²

b = coefficient of x

c = coefficient of the independent number

$$ x^2+4x-5=0 $$

What are the components of the equation?

Question 2

a = coefficient of x²

b = coefficient of x

c = coefficient of the independent number

$$ x^2+7x=0 $$

What are the components of the equation?

Question 3

a = Coefficient of x²

b = Coefficient of x

c = Coefficient of the independent number

what is the value of $$ a $$ in the equation

$$ y=3x-10+5x^2 $$

Examples with solutions for Solving Quadratic Equations

Exercise #1

Solve the following:

$x^2+5x+4=0$

Video Solution

Step-by-Step Solution

Notice that the quadratic equation:

$x^2+5x+4=0 =0$

and this is because there is a quadratic term (meaning raised to the second power),

The first step in solving a quadratic equation is always arranging it in a form where all terms on one side are ordered from highest to lowest power (in descending order from left to right) and 0 on the other side,

Then we can choose whether to solve it using the quadratic formula or by factoring/completing the square.

The equation in the problem is already arranged, so let's proceed with the solving technique:

We'll choose to solve it using the quadratic formula,

Let's recall it first:

The rule states that the roots of an equation of the form:

$ax^2+bx+c=0$

are:

$x_{1,2}=\frac{-b\pm\sqrt{b^2-4ac}}{2a}$

(meaning its solutions, the two possible values of the unknown for which we get a true statement when substituted in the equation)

This formula is called: "The Quadratic Formula"

Let's return to the problem:

$x^2+5x+4=0 =0$

And solve it:

First, let's identify the coefficients of the terms:

$\begin{cases}a=1 \\ b=5 \\ c=4\end{cases}$

where we noted that the coefficient of the quadratic term is 1,

And we'll get the solutions of the equation (its roots) by substituting the coefficients we just identified into the quadratic formula:

$x_{1,2}=\frac{-b\pm\sqrt{b^2-4ac}}{2a}=\frac{-5\pm\sqrt{5^2-4\cdot1\cdot4}}{2\cdot1}$

Let's continue and calculate the expression inside the square root and simplify the expression:

$x_{1,2}=\frac{-5\pm\sqrt{9}}{2}=\frac{-5\pm3}{2}$

Therefore the solutions of the equation are:

$\begin{cases}x_1=\frac{-5+3}{2}=-1 \\ x_2=\frac{-5-3}{2}=-4\end{cases}$

Therefore the correct answer is answer C.

Answer

$x_1=-1,x_2=-4$

Exercise #2

Solve the following equation:

$2x^2-10x-12=0$

Video Solution

Step-by-Step Solution

Let's recall the quadratic formula:

$Quadratic formula | The formula$

We'll substitute the given data into the formula:

$x={{-(-10)\pm\sqrt{-10^2-4\cdot2\cdot(-12)}\over 2\cdot2}}$

Let's simplify and solve the part under the square root:

$x={{10\pm\sqrt{100+96}\over 4}}$

$x={{10\pm\sqrt{196}\over 4}}$

$x={{10\pm14\over 4}}$

Now we'll solve using both methods, once with the addition sign and once with the subtraction sign:

$x={{10+14\over 4}} = {24\over4}=6$

$x={{10-14\over 4}} = {-4\over4}=-1$

We've arrived at the solution: X=6,-1

Answer

$x_1=6$ $x_2=-1$

Exercise #3

Solve the following equation:

$x^2+3x-18=0$

Video Solution

Step-by-Step Solution

Notice that the quadratic equation:

$x^2+3x-18=0$

and this is because there is a quadratic term (meaning raised to the second power),

Then we can choose whether to solve it using the quadratic formula or by factoring/completing the square.

The equation in the problem is already arranged, so let's proceed with the solving technique:

We'll choose to solve it using the quadratic formula,

Let's recall it first:

The rule states that the roots of the equation of the form:

$ax^2+bx+c=0$

are:

$x_{1,2}=\frac{-b\pm\sqrt{b^2-4ac}}{2a}$

(meaning its solutions, the two possible values of the unknown for which we get a true statement when substituted in the equation)

This formula is called: "The Quadratic Formula"

Let's return to the problem:

$x^2+3x-18=0$

And solve it:

First, let's identify the coefficients of the terms:

$\begin{cases}a=1\\b=3\\c=-18\end{cases}$

where we noted that the coefficient of the quadratic term is 1,

And we'll get the solutions of the equation (its roots) by substituting the coefficients we just noted in the quadratic formula:

$x_{1,2}=\frac{-b\pm\sqrt{b^2-4ac}}{2a}=\frac{-3\pm\sqrt{3^2-4\cdot1\cdot(-18)}}{2\cdot1}$

Let's continue and calculate the expression inside the square root and simplify the expression:

$x_{1,2}=\frac{-3\pm\sqrt{81}}{2}=\frac{-3\pm9}{2}$

Therefore the solutions of the equation are:

$\begin{cases}x_1=\frac{-3+9}{2}=3 \\ x_2=\frac{-3-9}{2}=-6\end{cases}$

Therefore the correct answer is answer C.

Answer

$x_1=3,x_2=-6$

Exercise #4

Solve the following equation:

$x^2+5x+4=0$

Video Solution

Step-by-Step Solution

The parameters are expressed in the quadratic equation as follows:

aX2+bX+c=0

We substitute into the formula:

-5±√(5²-4*1*4)
2

-5±√(25-16)
2

-5±√9
2

-5±3
2

The symbol ± means that we have to solve this part twice, once with a plus and a second time with a minus,

This is how we later get two results.

-5-3 = -8
-8/2 = -4

-5+3 = -2
-2/2 = -1

And thus we find out that X = -1, -4

Answer

$x_1=-1$ $x_2=-4$

Exercise #5

Solve the following equation:

$x^2+5x+6=0$

Video Solution

Step-by-Step Solution

Notice that the quadratic equation:

$x^2+5x+6=0$

and this is because there is a quadratic term (meaning raised to the second power),

Then we can choose whether to solve it using the quadratic formula or by factoring/completing the square.

The equation in the problem is already arranged, so let's proceed with the solving technique:

We'll choose to solve it using the quadratic formula,

Let's recall it first:

The rule states that the roots of an equation of the form:

$ax^2+bx+c=0$

are:

$x_{1,2}=\frac{-b\pm\sqrt{b^2-4ac}}{2a}$

(meaning its solutions, the two possible values of the unknown for which we get a true statement when substituted in the equation)

This formula is called: "The Quadratic Formula"

Let's return to the problem:

$x^2+5x+6=0$ and solve it:

First, let's identify the coefficients of the terms:

$\begin{cases}a=1 \\ b=5 \\ c=6\end{cases}$

where we noted that the coefficient of the quadratic term is 1,

And we'll get the equation's solutions (roots) by substituting the coefficients we just noted into the quadratic formula:

$x_{1,2}=\frac{-b\pm\sqrt{b^2-4ac}}{2a}=\frac{-5\pm\sqrt{5^2-4\cdot1\cdot6}}{2\cdot1}$

Let's continue and calculate the expression inside the square root and simplify the expression:

$x_{1,2}=\frac{-5\pm\sqrt{1}}{2}=\frac{-5\pm1}{2}$

Therefore the solutions to the equation are:

$\begin{cases}x_1=\frac{-5+1}{2}=-2 \\ x_2=\frac{-5-1}{2}=-3\end{cases}$

Therefore the correct answer is answer D.

Answer

$x_1=-3,x_2=-2$

Start practice

Methods for Solving a Quadratic Function