Sum of Fractions

In this article, you will learn the easiest ways to add fractions, this will allow you to add all kinds of fractions without any inconvenience.
Shall we start?

The first step to solving a fraction addition is to find the common denominator.
Getting a common denominator means that we end up with two fractions with the same denominator. We will do this by simplifying, expanding, or multiplying the denominators.
After finding the common denominator, we will continue with the second step.
The second step to solving a fraction addition is to add the numerators.
We may encounter different cases of additions that we will study next:

One of the denominators that appear in the initial exercise will be the common denominator.

Sometimes we will encounter quite simple fractions where we won't need to expand or simplify both, but only one of the fractions.
Let's see an example:

$\frac{3}{2}+\frac{1}{4}=$

Upon observing these denominators, we will immediately realize that, if we multiply the denominator $2$ by $2$, we will reach the denominator $4$.
This way, we will reach the common denominator and will be able to solve the exercise easily.

Observe -> When multiplying the denominator to transform it into the common denominator, we must also multiply the numerator by the same number so that the value of the fraction does not change.

We will do this by expanding by $2$ and we will obtain:

$\frac{6}{4}+\frac{1}{4}=$

Now let's move to the second step and add the numerators.
Attention –> We do not add the denominators. Once we reach the common denominator, only the numerators are added, which, in fact, have the same denominator.

Let's see it in an exercise:

$\frac{6}{4}+\frac{1}{4}=\frac{7}{4}$

We add $1+6$ and leave the denominator only once.

If we wish, we can simplify the fraction and write it as follows: $1\frac{3}{4}$

Another exercise:

$\frac{1}{3}+\frac{2}{6}=$

Solution:
We will realize that, if we multiply $3$ by $2$ we will reach $6$ and, this will be the common denominator.

We will obtain:

$​​\frac{2}{6}+\frac{2}{6}=$

Let's add the numerators and we will obtain:
$\frac{4}{6}$
We can simplify and arrive at: $\frac{2}{3}$

The common denominator will be the product of the given denominators.

Sometimes we will encounter slightly more complicated exercises in which it will not be enough to expand a single fraction to obtain the common denominator, but rather, we must intervene in both fractions.
Do not worry, the way to act in such a case is simply to multiply the first fraction by the denominator of the second and multiply the second fraction by the denominator of the first.

See how simple this is:

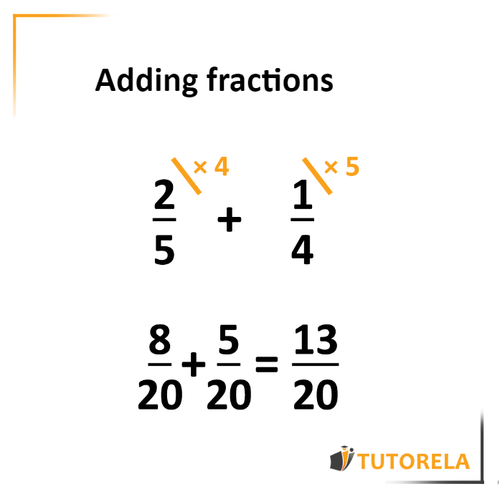
Let's multiply the denominators:
We will multiply the $\frac{2}{5}$ by $4$ (the denominator of the second fraction) and the $\frac{1}{4}$ by $5$ (the denominator of the first fraction).
We will obtain:
$\frac{8}{20}+\frac{5}{20}=$

Let's add the numerators and we will arrive at the solution:
$\frac{13}{20}$
Did you see how simple it was? This is a technical method that does not require us to think about how to reach the common denominator.
Therefore, we recommend using it in all fraction addition exercises.

Sum of $3$ fractions

In case there are $3$ fractions with different denominators in the exercise, we will first find a common denominator for $2$ of them (the simplest ones), then we will find the common denominator between the one we obtained and the third given fraction.

Let's see an example and you'll see it's very simple:
$\frac{2}{10}+\frac{2}{3}+\frac{4}{5}=$
Let's look at the denominators and ask ourselves - Among the three denominators, which pair of them is easier to find a common denominator for?
The answer is $5$ and $10$, since $10$ is the common denominator for both.
Therefore, we will multiply $\frac{4}{5}$ by $2$ and we will get:
$\frac{2}{10}+\frac{2}{3}+\frac{8}{10}=$
Now we can add the numerators that already have a common denominator to arrive at a clearer and more orderly exercise (this step is not mandatory, but it will help us later.

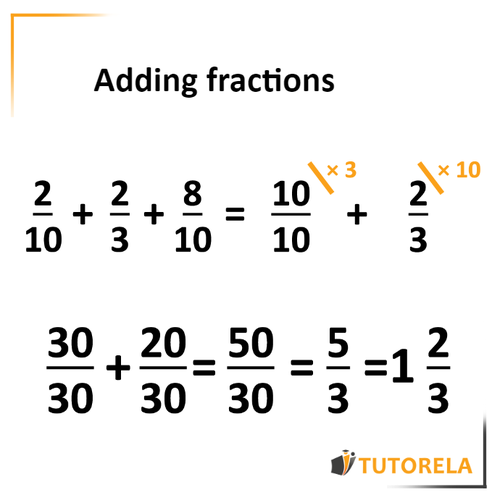
Now we just have to find the common denominator between $10$ -> the new denominator we found, and $3$ the third denominator of the exercise.
We will do this with the method of multiplying denominators and we will get:
$\frac{30}{30}+\frac{20}{30}=$
Let's add the numerators and we will get:
$\frac{50}{30}$
We can simplify and arrive at:
$\frac{5}{3}=1\frac{2}{3}$