The Parabola
This function is a quadratic function and is called a parabola.
We will focus on two main types of parabolas: maximum and minimum parabolas.
This function is a quadratic function and is called a parabola.
We will focus on two main types of parabolas: maximum and minimum parabolas.
Also called smiling or happy.
A vertex is the minimum point of the function, where is the lowest.
We can identify that it is a minimum parabola if the equation is positive.

Also called sad or crying.
A vertex is the maximum point of the function, where is the highest.
We can identify that it is a maximum parabola if the equation is negative.
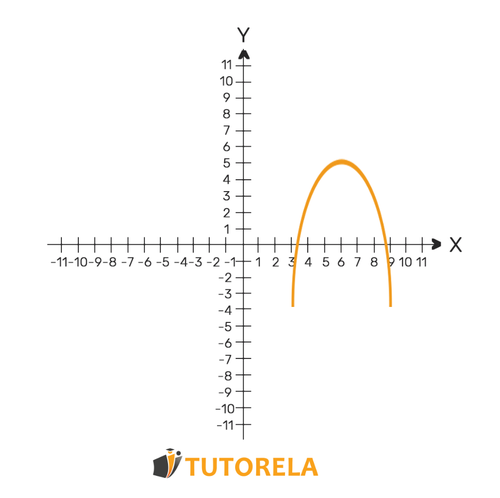
To the parabola,
the vertex marks its highest point.
How do we find it?
What is the value of the coefficient \( c \) in the equation below?
\( 3x^2+5x \)
One of the following two methods can be chosen:
The value of that we receive will be replaced in the parabola function and we will obtain the value of relevant.
What is the value of the coefficient \( b \) in the equation below?
\( 3x^2+8x-5 \)
What is the value of the coefficient \( c \) in the equation below?
\( 4x^2+9x-2 \)
Identify the coefficients based on the following equation
\( y=-5x^2 \)
The formula to find a vertex using two symmetric points is:
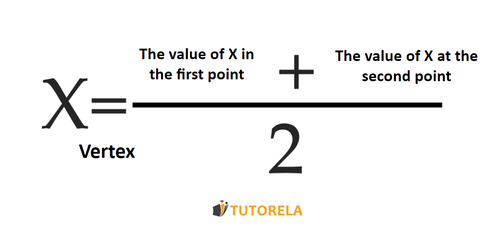
The vertex that we receive in the function to find the value of the vertex .
Now, we will move on to the points of intersection of the parabola with the and axes
When we want to find the point of intersection with the axis:
We will set in the quadratic equation and solve using a trinomial or the root formula.
We can find parabolas that are not zero and that do not have any point of intersection with the axis, or that have or a maximum of .
When we want to find a point of intersection with the axis:
We will set in the quadratic equation and find the solutions.
Wonderful. Now we will move on to the areas of increase and decrease of the quadratic function.
\( y=2x^2+3 \)
Identify the coefficients based on the following equation
\( y=-2x^2+3x+10 \)
Identify the coefficients based on the following equation
\( y=x^2 \)
The areas of increase and decrease describe the where the parabola increases and where the parabola decreases.
The parabola changes its domain once, at the vertex.
Let's see this in the figure:

When there is a graph:
We will examine what happens when the s are smaller than the vertex and what happens when the s are greater than the vertex .
When there is no graph:
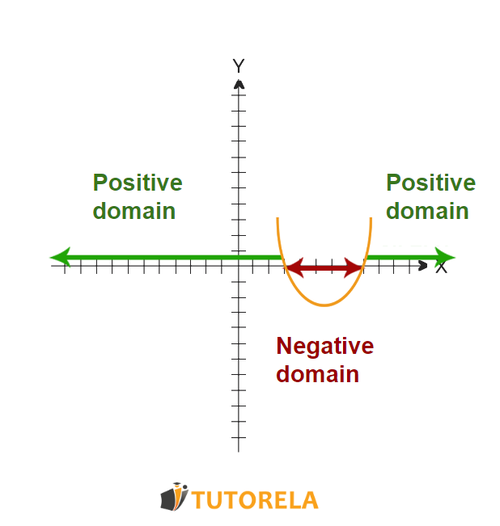
Positive domain: describes the where the graph of the parabola is above the axis, with a value positive.
Negative domain: describes the where the graph of the parabola is below the axis, with a negative value.
To find the domains of positivity and negativity, we will plot the graph of the parabola and ask:
At what values is the graph of the parabola above the axis, with a positive value? This will be the domain of positivity of the parabola.
At what values is the graph of the parabola below the axis, with a negative value? This will be the domain of negativity of the parabola.
Let's see this on the graph:
Here we have a quadratic equation.
A quadratic equation is always constructed like this:
Where a, b, and c are generally already known to us, and the X and Y points need to be discovered.
Firstly, it seems that in this formula we do not have the C,
Therefore, we understand it is equal to 0.
a is the coefficient of X², here it does not have a coefficient, therefore
is the number that comes before the X that is not squared.
In fact, a quadratic equation is composed as follows:
y = ax²-bx-c
That is,
a is the coefficient of x², in this case 2.
b is the coefficient of x, in this case 5.
And c is the number without a variable at the end, in this case 6.
Identify the coefficients based on the following equation
Let's determine the coefficients for the quadratic function given by .
Comparing these coefficients to the provided choices, the correct answer is:
.
Therefore, the correct choice is Choice 4.
Identify the coefficients based on the following equation
The given quadratic function is .
To identify the coefficients, let's compare this with the standard form of a quadratic equation, .
Thus, we have identified the coefficients as , , and .
Therefore, the correct answer is .
The correct choice is
Determine the value of the coefficient in the following equation:
The quadratic equation in the problem is already arranged (meaning all terms are on one side and 0 on the other side), so let's proceed to answer the question asked:
The question asked in the problem - What is the value of the coefficient in the equation?
Let's recall the definitions of coefficients in solving quadratic equations and the roots formula:
The rule states that the roots of an equation of the form:
are:
That is the coefficient is the coefficient of the quadratic term (meaning the term with the second power)- Let's examine the equation in the problem:
Remember that the minus sign before the quadratic term means multiplication by: , therefore- we can write the equation as:
The number that multiplies the , is hence we identify that the coefficient of the quadratic term is the number ,
Therefore the correct answer is A.
-1
\( y=x^2+10x \)
Identify the coefficients based on the following equation
\( y=-x^2+x+5 \)
Identify the coefficients based on the following equation
\( y=x^2+x+5 \)