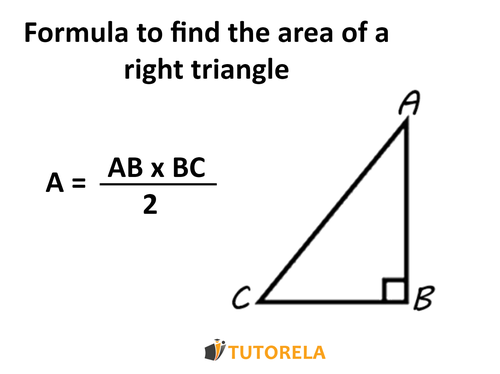
To find the area of the triangle, we will use the formula for the area of a triangle:
Area=21×base×height
From the problem:
- The length of the base BC is given as 7 units.
- The height from point A perpendicular to the base BC is given as 4.5 units.
Substitute the given values into the area formula:
Area=21×7×4.5
Calculate the expression step-by-step:
Area=21×31.5
Area=15.75
Therefore, the area of the triangle is 15.75 square units. This corresponds to the given choice: 15.75.