Representing a Function Verbally and with Tables

Function, describes a correlation or coincidence between a dependent variable ( $Y$ ) and an independent variable ( $X$ ). The legitimacy of this relationship between the variables is called the " correspondence rule ".

Verbal representation of a function

The verbal representation of a function expresses the connection between variables verbally, i.e. through a story.

A typical verbal representation of a function can look like this:

Assuming that Daniel reads all the books he buys that month, the total number of books Daniel reads per year ( $Y$ ) is a function of the number of books Danny buys each month ( $X$ ).

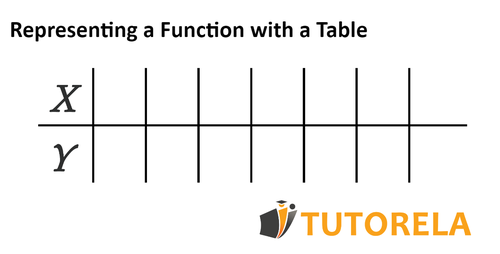
Tabular representation of a function

A tabular representation of a function is a demonstration of the legitimacy of a function using a table of values $X$ (independent variable) and the corresponding values $Y$ (dependent variable).

In general, a table of values is shown as follows:

A1 - Verbal representation of a new function

Detailed explanation

Start practice

Test yourself on representations of functions!

Determine whether the data in the following table represent a constant function

Practice more now

Examples of exercises on verbal and tabular representation of a function

Example 1

$Y$ is a function of $X$ that corresponds to any value of $X$ a number less than it in $2$ .

$Y=X-2$

Solve the equation for each of the numbers of $X$ represented in the following table and place the correct number in. $Y$ .

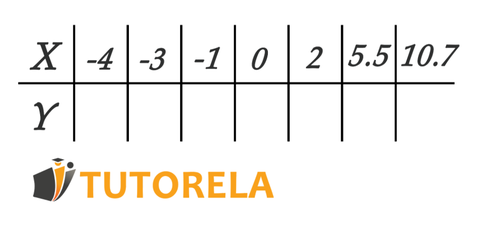
verbal and tabular representation of a function

If $X = 1$ , then Y will be equal to ____________

If $X = 13$ , then Y will equal ___________ .

A value of $X$ corresponding to $Y = 10,5$ is __________

A value $X$ corresponding to $Y = 0$ is __________

Join Over 30,000 Students Excelling in Math!

Endless Practice, Expert Guidance - Elevate Your Math Skills Today

Test your knowledge

Question 1

Determine whether the following table represents a function

Question 2

Determine whether the following table represents a function

Question 3

Determine whether the following table represents a function

Practice of verbal and tabular representation of a function

Example 2

Describe in words the relationship between $X$ e $Y$ .

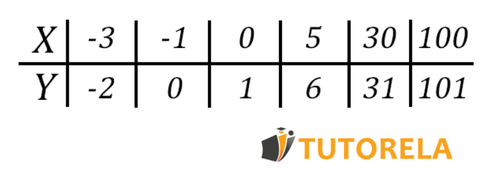
Describe in words the relationship between X and Y.

Example 3

Write down which table represents a function and which table does not represent a function

Do you know what the answer is?

Question 1

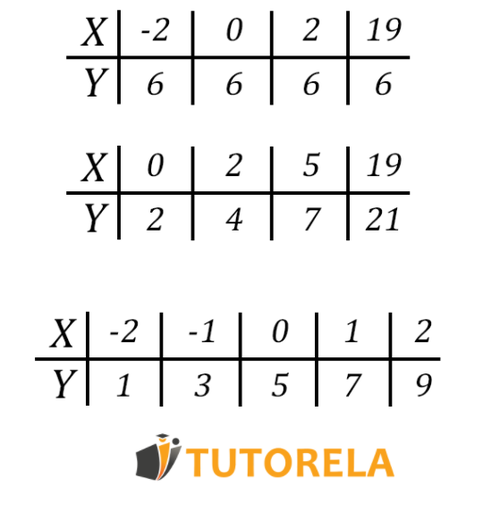
Determine whether the following table represents a function

Question 2

Does the graph below represent a function?

Question 3

Is the given graph a function?

Example 4

$Y$ is a function of $X$ that corresponds to any value of $X$ a number that is $5$ times greater than it.

$Y=5X$

Complete the table of values

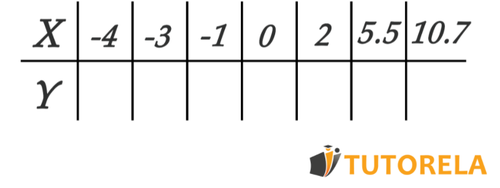
a function of X corresponding to any value of X

If $X = 1$ , then $Y$ will equal ____________
If $X = 13$ , then $Y$ will be equal to ____________
If $Y = 10,5$ , then $X$ will be equal to ____________
If $Y = 0$ , then $X$ will be equal to ____________

Example 5

$Y$ is a function of $X$ that corresponds to any value of $X$ a number $4$ times less than it.

$Y=\frac{X}{4}$

Complete the table of values

If $X = 1$ , then $Y$ will be equal to ____________
If $X = 13$ , then $Y$ will be equal to ____________
If $Y = 10,5$ , then $X$ will be equal to ____________
If $Y = 0$ , then $X$ will be equal to ____________

Check your understanding

Question 1

Is the given graph a function?

Question 2

Is the given graph a function?

Question 3

Is the given graph a function?

Example 6

Complete the following table

If $X = 10$ , then $Y$ will equal ____________
If $X = -2$ , then $Y$ will be equal to ____________
If $Y = 100$ , then $X$ will be equal to ____________
If $Y = -20$ , then $X$ will be equal to ____________

Example 7

Answer the following questions (for each example, write a table of values and draw the graph)

Write an example of a function whose graph describes it as a continuous graph.
Write an example of a function whose graph is discrete.

Do you think you will be able to solve it?

Question 1

Is the given graph a function?

Question 2

Is the given graph a function?

Question 3

Determine whether the following table represents a function

Example 8

The function $Y$ corresponds to any number $X$ that is its root.

$Y=\sqrt{X}$

Complete the table of values

If $X = 1$ , then $Y$ will be equal to ____________
If $X = 13$ , then $Y$ will be equal to ____________
If $Y = 10,5$ , then $X$ will be equal to ____________
If $Y = 0$ , then $X$ will be equal to ____________

Example 9

The function corresponds to any number $X$ less than $3$ of half the number

$Y=\frac{X}{2}-3$

Complete the table of values

If $X = 1$ , then $Y$ will be equal to ____________
If $X = 13$ , then $Y$ will be equal to ____________
If $Y = 10,5$ , then $X$ will be equal to ____________
If $Y = 0$ , then $X$ will be equal to ____________

Test your knowledge

Question 1

Given the following graph, determine which table corresponds to the following table

Question 2

Given the following graph, determine which table corresponds to the following table

Question 3

Determine whether the data in the following table represent a constant function

Example 10

The function $Y$ corresponds to any number $X$ greater in $5$ times the number

$Y=2X+5$

Complete the table of values

If $X = 1$ , then $Y$ will be equal to ____________
If $X = 13$ , then $Y$ will be equal to ____________
If $Y = 10,5$ , then $X$ will be equal to ____________
If $Y = 0$ , then $X$ will be equal to ____________

Review questions

What is a function?

A function is a relationship between two variables, the variable $Y$ which is called the dependent variable, and the variable $X$ , which is called the independent variable, between these two variables there is a correspondence rule; that is, for each value of $X$ there is only one value of $Y$ .

What are the ways of representing a relationship?

A function can be represented as follows:

Verbally
Algebraically
Table of values (Tabular)
Graphically.

How to represent functions step by step?

Let's see an example of how to represent a function.

Example:

Represent the following function in its different forms

Verbally:

Let $Y$ be a function of $X$ such that a value of $X$ corresponds to a number increased by $4$

Algebraically:

$Y=X+4$

Table of values:

We already have the function in algebraic form, now we are going to give values to $X$ , to find the value of $Y$ , according to the correspondence rule, and these values we are going to register them in a table:

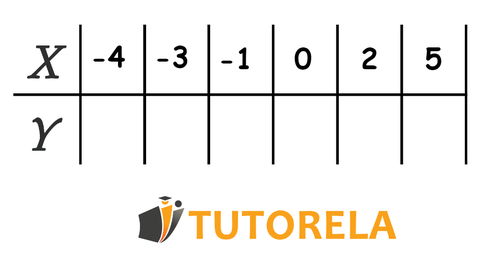
1 - How to represent functions step by step

Now we are going to substitute the values of $X$ , to register the value that corresponds to $Y$ , let's start with

We already know that the algebraic expression of this function is:

$Y=X+4$

Then,

When $X=-4$

$Y=-4+4=0$

When $X=-3$

$Y=-3+4=1$

When $X=-1$

$Y=-1+4=3$

When $X=0$

$Y=0+4=4$

When $X=2$

$Y=2+4=6$

When $X=5$

$Y=5+4=9$

According to this data we are now going to record it in the table

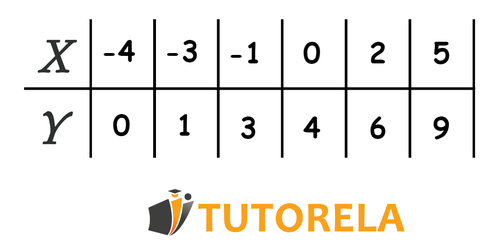
2 - How to represent functions step by step

We have represented the function in a table of values.

Graphically:

Finally we are going to represent these values in a graph:

We are going to find the pairs of coordinates in the Cartesian plane, and join each point as follows.

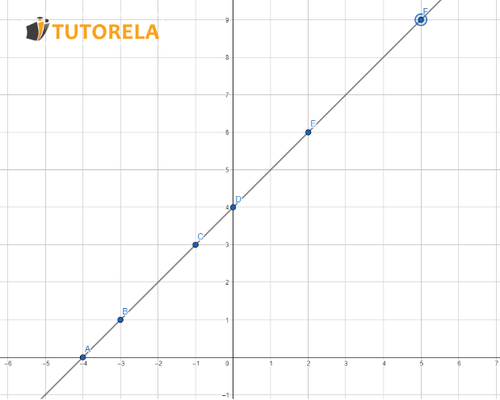
3 - How to represent functions step by step

We can see that the function in graphical form is a linear function because it forms a straight line.

If you are interested in this article you may also be interested in the following articles:

Graphical representation of a function

Algebraic representation of a function

Notation of a function

Domain of a function

Indefinite integral

Numerical value assignment in a function

Increasing function

Decreasing function

Constant function

Growing and decreasing intervals of a function

Functions for seventh grade

In Tutorela you will find a variety of articles with interesting explanations about mathematics.

Do you know what the answer is?

Question 1

Determine whether the following table represents a function

Question 2

Determine whether the following table represents a function

Question 3

Determine whether the following table represents a function

Examples with solutions for Representing a Function Verbally and with Tables

Exercise #1

Determine whether the data in the following table represent a constant function

Video Solution

Step-by-Step Solution

It should be remembered that a constant function describes a situation where as the X value increases, the function value (Y) remains constant.

In the table, we can see that there is a constant change in X values, meaning an increase of 1, and a non-constant change in Y values - sometimes increasing by 1 and sometimes by 4

Therefore, according to the rule, the table does not describe a function

Answer

Exercise #2

Determine whether the following table represents a function

Video Solution

Step-by-Step Solution

It is important to remember that a constant function describes a situation where as the X value increases, the function value (Y) remains constant.

In the table, we can see that there is a constant change in X values, meaning an increase of 1, and a constant change in Y values, meaning an increase of 3

Therefore, according to the rule, the table describes a function.

Answer

Yes

Exercise #3

Determine whether the following table represents a function

Video Solution

Step-by-Step Solution

It is important to remember that a constant function describes a situation where as the X value increases, the function value (Y) remains constant.

In the table, we can see that there is a constant change in the X values, specifically an increase of 2, and the Y value remains constant.

Therefore, according to the rule, the table describes a constant function.

Answer

Yes

Exercise #4

Does the graph below represent a function?

Video Solution

Step-by-Step Solution

It is important to remember that a function is an equation that assigns to each value in domain $x$ only one value in range $y$ .

Since we can see that for every $x$ value found on the graph there is only one corresponding $y$ value, the graph is indeed a function.

Answer

Yes

Exercise #5

Is the given graph a function?

Video Solution

Step-by-Step Solution

It is important to remember that a function is an equation that assigns to each element in domain X one and only one element in range Y

We should note that for every X value found on the graph, there is one and only one corresponding Y value.

Therefore, the graph is indeed a function.

Answer

Yes

Start practice

Representing a Function Verbally and with Tables

Verbal representation of a function

Tabular representation of a function

Test yourself on representations of functions!

Example 1

Practice of verbal and tabular representation of a function

Example 2

Example 3

Example 4

Example 5

Y=X4 Y=\frac{X}{4} Y=4X​

Example 6

Example 7

Example 8

Y=X Y=\sqrt{X} Y=X​

Example 9

Y=X2−3 Y=\frac{X}{2}-3 Y=2X​−3

Example 10

Y=2X+5 Y=2X+5 Y=2X+5

Review questions

Examples with solutions for Representing a Function Verbally and with Tables

Exercise #1

Video Solution

Step-by-Step Solution

Answer

Exercise #2

Video Solution

Step-by-Step Solution

Answer

Exercise #3

Video Solution

Step-by-Step Solution

Answer

Exercise #4

Video Solution

Step-by-Step Solution

Answer

Exercise #5

Video Solution

Step-by-Step Solution

Answer

More Questions

Representations of Functions

$Y=\frac{X}{4}$

$Y=\sqrt{X}$

$Y=\frac{X}{2}-3$

$Y=2X+5$