How to calculate the area of a triangle using trigonometry?

Throughout your geometry studies, which deal with different structures and shapes, you are required to calculate areas and perimeters. As known, each shape or structure has a different formula through which you can answer the question and calculate the area. Fortunately, there is one formula that you can use for all triangles, and it can answer your question about how to calculate the area of a triangle using trigonometry.

In mathematics studies, emphasis is also placed on trigonometry, which deals with the study of triangles, their angles and sides. Both students studying in level B math in middle school, and those who take 3 units in high school, are required to demonstrate knowledge of triangles (from right triangles to isosceles triangles), and thus also answer the question of how to calculate the area of a triangle using trigonometry.

Now that you know the formula for calculating the area of a triangle using trigonometry, you can use it in any question where you need to calculate areas in triangles. The formula for calculating the triangle:

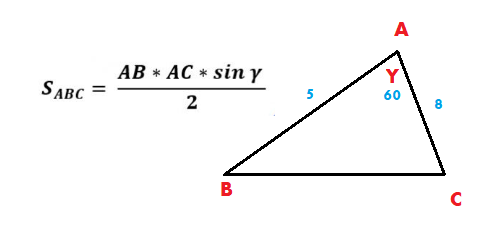
Example:

Given triangle $ABC$ and it is known that:

Side $AB$ equals $5$

Side $AC$ equals $8$

Angle $Y$ is $60$ degrees.

Let's substitute the given values into the formula and we'll get:

$s =\frac {AC \cdot AB \cdot \sin60} {2}$

In other words:

$s =\frac {5\cdot 8\cdot 0.866} {2}$

The result obtained is: $17.32$.