Area of Isosceles Triangles

🏆Practice triangle area
5 Questions
Triangle
Triangle area
Practice
Add a new subject

Formula to calculate the area of an isosceles triangle

Height of the base × Base2=A \frac{Height~of~the~base~\times ~Base}{2}=A

Start practice

Test yourself on triangle area!

einstein

Calculate the area of the triangle ABC using the data in the figure.

121212888999AAABBBCCCDDD

Practice more now

Area of Isosceles Triangles

Calculating the area of an isosceles triangle is very simple, easy, and even identical to the calculation we do to find out the area of other types of triangles. Therefore, if you happen to get a question about calculating the area of isosceles triangles on the exam, I assure you that a small smile will appear on your face.

How is the area of an isosceles triangle calculated?

We will multiply the base by the height and divide by two.

Remember!

The main property of the isosceles triangle is that the median of the base, the bisector, and the height are the same, that is, they coincide. Therefore, even if the question only names the median of the base or the bisector, you can immediately deduce that it is also the height of the triangle and use it to calculate its area.

Observe the theorem holds true only with the height, the median of the base, and the bisector!

You didn't think we were going to send you off without any exercises on the topic, did you? Time to practice!

Join Over 30,000 Students Excelling in Math!
Endless Practice, Expert Guidance - Elevate Your Math Skills Today
Test your knowledge
Question 1

Calculate the area of the right triangle below:

101010666888AAACCCBBB

Question 2

Calculate the area of the following triangle:

666777AAABBBCCCEEE

Question 3

Calculate the area of the following triangle:

444444AAABBBCCCEEE

Let's start with a classic exercise

Here you have an isosceles triangle ABCABC

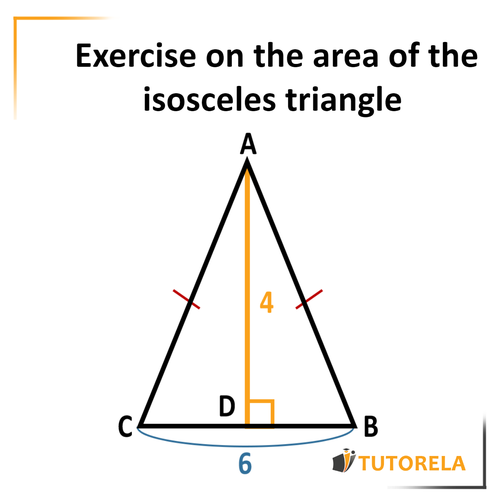
Given that:
ab=acab=ac
ADAD -

Height
AD=4AD = 4
CB=6CB=6

What is the area of the triangle?

Solution: We will proceed according to the formula - the height AD=4AD = 4
multiply by the base CB=6CB = 6
and divide the received product by 22
We will obtain:
4×62=12 \frac{4\times6}{2}=12
The area of the triangle ABCABC is 1212 cm2.

Now let's move on to an exercise that aims to be a bit more sophisticated:

You have the isosceles triangle FDCFDC

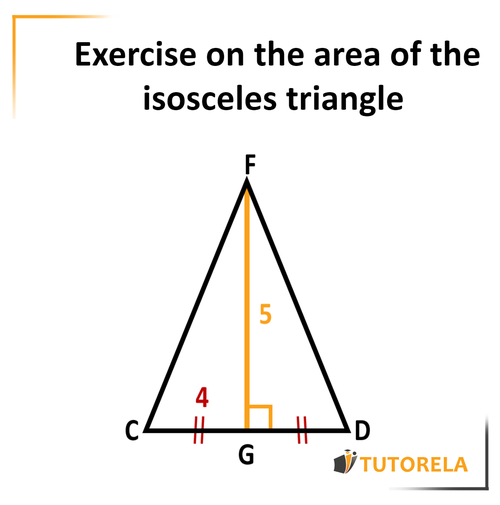
Given that:
FC=FDFC=FD
CG=4CG= 4
FG=5FG = 5 The median of the base

Calculate the area

Solution: Let's remember that, in an isosceles triangle, the median of the base is also the height, therefore, we can use it in the formula for the area of the isosceles triangle. Let's note: Height FG=5FG=5
Now let's see that we have only half of the base CG=4CG =4 .
Since FGFG is given as the median, we can deduce that also GB=4GB=4 and consequently, the entire side of the base CD=8CD=8
Now let's put it in the formula:
4×82=16\frac{4\times 8}{2}=16
The area of the triangle FDCFDC is 1616 cm2 .

Do you know what the answer is?
Question 1

Calculate the area of the following triangle:

444555AAABBBCCCEEE

Question 2

Calculate the area of the following triangle:

888101010AAABBBCCCEEE

Question 3

Calculate the area of the triangle using the data in the figure below.

888666AAABBBCCC10

Bonus exercise (tip) for advanced level

Formula to calculate the area of an isosceles triangle that is also a right triangle:

If you come across calculating the area of an isosceles triangle whose height has not been given, but you know it is a right triangle, it is useful to know the following trick:

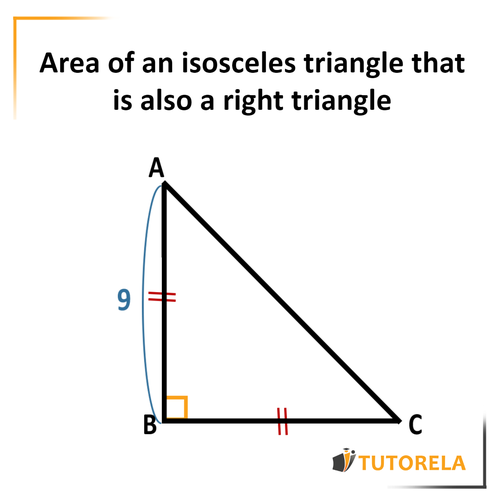
Let's see how it is done by applying it in an exercise: Before you, you have an isosceles right triangle ABCABC
Given that AB=ACAB=AC
angle ABC=90ABC = 90
AB=4AB=4

Calculate the area of the triangle

Solution: Let's not be scared of not having data about the height and proceed according to the formula: the triangle is isosceles, therefore AB=AC=3AB=AC=3.

These are the two legs of the triangle - they form a right angle. Consequently, we will obtain:
4×42=8 \frac{4\times4}{2}=8
The area of the triangle is 88 cm2 .

Examples and exercises with solutions for the area of an isosceles triangle

examples.example_title

Calculate the area of the triangle below, if possible.

8.58.58.5777

examples.explanation_title

The formula to calculate the area of a triangle is:

(side * height corresponding to the side) / 2

Note that in the triangle provided to us, we have the length of the side but not the height.

That is, we do not have enough data to perform the calculation.

examples.solution_title

Cannot be calculated

examples.example_title

Which of the following triangles have the same area?

101010121212555131313555888121212666666FFFEEEGGGCCCBBBAAAKKKJJJIIIDDDLLLHHH

examples.explanation_title

We calculate the area of triangle ABC:

12×52=602=30 \frac{12\times5}{2}=\frac{60}{2}=30

We calculate the area of triangle EFG:

6×102=602=30 \frac{6\times10}{2}=\frac{60}{2}=30

We calculate the area of triangle JIK:

6×52=302=15 \frac{6\times5}{2}=\frac{30}{2}=15

It can be seen that after the calculation, the areas of the similar triangles are ABC and EFG

examples.solution_title

EFG, ABC

examples.example_title

Given the triangle PRS

The length of side SR is 4 cm

The area of the triangle PSR is 30 cm²

Calculate the height PQ

S=30S=30S=30444PPPRRRSSSQQQ

examples.explanation_title

We use the formula to calculate the area of the triangle.

Pay attention: in the obtuse triangle, its height is located outside the triangle!

Lado⋅Altura2=Aˊrea del triangulo \frac{Lado\cdot\text{Altura}}{2}=Área~del~triangulo

Double the equation by a common denominator.

4⋅PQ2=30 \frac{4\cdot PQ}{2}=30

⋅2 \cdot2

Divide the equation by the coefficient of PQ PQ .

4PQ=60 4PQ=60 / :4 :4

PQ=15 PQ=15

examples.solution_title

15 cm

examples.example_title

Calculate X using the data in the figure below.

S=20S=20S=20555XXXAAABBBCCC

examples.explanation_title

The formula to calculate the area of a triangle is:

(side * height descending from the side) /2

We place the data we have into the formula to find X:

20=AB×AC2 20=\frac{AB\times AC}{2}

20=x×52 20=\frac{x\times5}{2}

Multiply by 2 to get rid of the fraction:

5x=40 5x=40

Divide both sections by 5:

5x5=405 \frac{5x}{5}=\frac{40}{5}

x=8 x=8

examples.solution_title

8

examples.example_title

The area of trapezoid ABCD is X cm².

The line AE creates triangle AED and parallelogram ABCE.

It is known that the ratio between the area of triangle AED and the area of parallelogram ABCE is 1:3.

Calculate the ratio between sides DE and EC

AAABBBCCCDDDEEE

examples.explanation_title

To calculate the ratio between the sides we will use the existing figure:

AAEDAABCE=13 \frac{A_{AED}}{A_{ABCE}}=\frac{1}{3}

We calculate the ratio between the sides according to the formula to find the area and then replace the data.

We know that the area of triangle ADE is equal to:

AADE=h×DE2 A_{ADE}=\frac{h\times DE}{2}

We know that the area of the parallelogram is equal to:

AABCD=h×EC A_{ABCD}=h\times EC

We replace the data in the formula given by the ratio between the areas:

12h×DEh×EC=13 \frac{\frac{1}{2}h\times DE}{h\times EC}=\frac{1}{3}

We solve by cross multiplying and obtain the formula:

h×EC=3(12h×DE) h\times EC=3(\frac{1}{2}h\times DE)

We open the parentheses accordingly

h×EC=1.5h×DE h\times EC=1.5h\times DE

We divide both sides by h

EC=1.5h×DEh EC=\frac{1.5h\times DE}{h}

We simplify to h

EC=1.5DE EC=1.5DE

Therefore, the ratio betweenECDE=11.5 \frac{EC}{DE}=\frac{1}{1.5}

examples.solution_title

1:1.5 1:1.5

Check your understanding
Question 1

Calculate the area of the triangle using the data in the figure below.

101010999AAABBBCCC

Question 2

Calculate the area of the triangle using the data in the figure below.

101010222AAABBBCCC

Question 3

Calculate the area of the triangle using the data in the figure below.

666888AAABBBCCC

Start practice
Related Subjects
👉  Click here  to practice